I’m trying to understand “In particular, we may identify $p$-colimits of $F$ with $p$-colimits of $F_0$.” 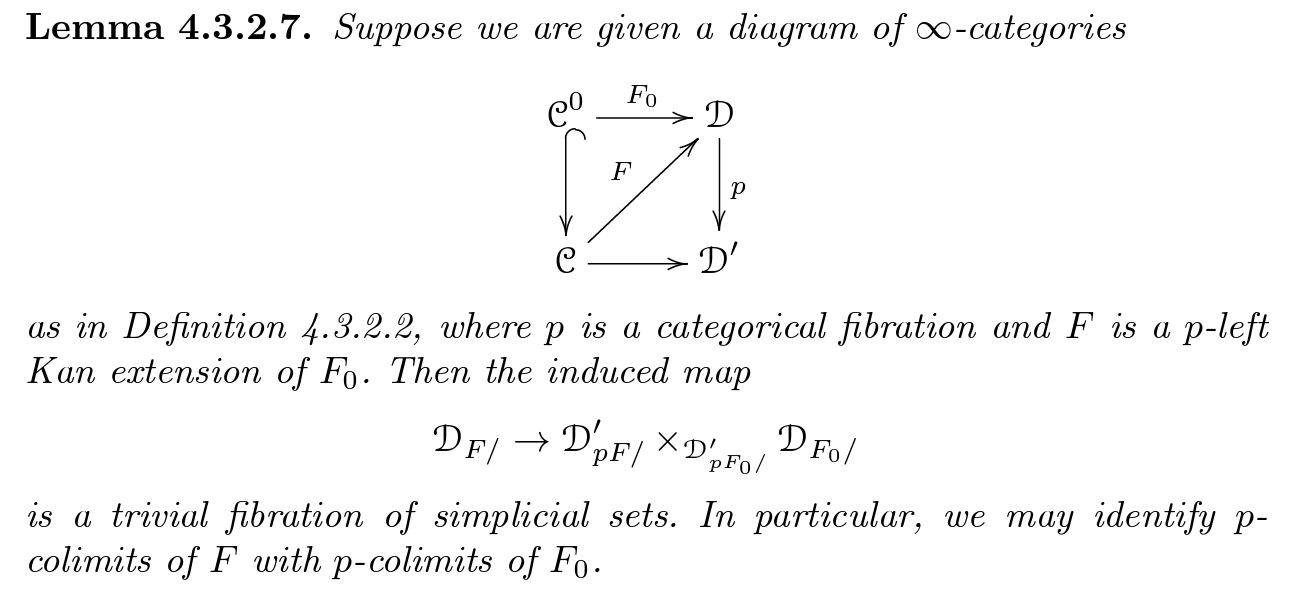It seems that here we have $C\rightarrow C*\mathrm{pt}$ is a homotopy pushout of right cone $C_0\rightarrow C_0*\mathrm{pt}$ via the embedding of quasi-categories $C_0\rightarrow C$, but why?

Or, is there another way to figure out “In particular, we may identify $p$-colimits of $F$ with $p$-colimits of $F_0$.”?