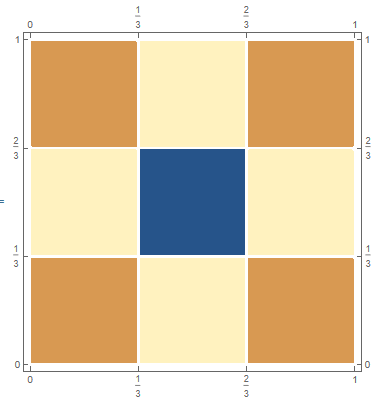
The answer is no. Indeed, partition the unit square $[0,1)^2$ into $9$ congruent squares as shown here:
Suppose that the joint pdf of $(X,Y)$ takes the constant value $3/4$ on each of the four dark-brown squares; the constant value $3/2$ on each of the four light-brown squares; and the constant value $0$ on the blue square.
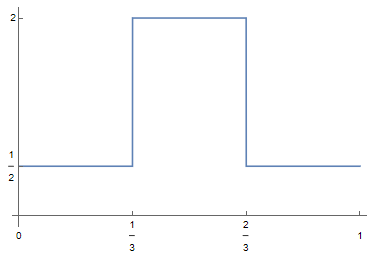
Then $X$ and $Y$ are each uniformly distributed on $[0,1]$, and hence their densities are nonincreasing on $[0,1]$, whereas $M=\min(X,Y)$ has the non-monotonic density whose graph is shown here: