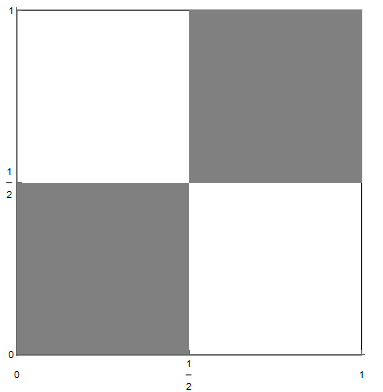
The answer is no. Indeed, let $X\sim U(0,1)$, where $U(0,1)$ is the uniform distribution on $[0,1]$, and let $Z$ be an independent copy of $X$. Next, let $$Y:=\frac Z2\,1(X<1/2)+\frac{1+Z}2\,1(X>1/2).$$ So, the distribution of the random point $(X,Y)$ is the half-and-half mixture of the uniform distribution on the square $(0,1/2)^2$ and the uniform distribution on the square $(1/2,1)^2$. That is, the distribution of the random point $(X,Y)$ is the uniform distribution on the union of the "gray" squares $(0,1/2)^2$ and $(1/2,1)^2$, shown here:
Then $Y\sim U(0,1)$. So, the common density of $X$ and $Y$ is nonincreasing on $[0,1]$.
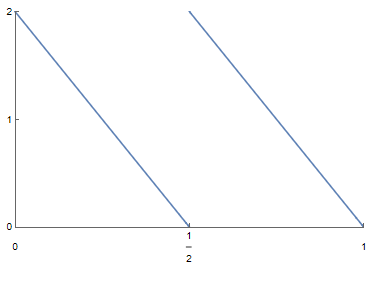
One the other hand, $M=\min(X,Y)$ has the non-monotonic density $g$ on $[0,1]$ such that $g(m)=2-4m$ for $m\in(0,1/2)$ and $g(m)=4-4m$ for $m\in(1/2,1)$.
Here is the graph of $g$:
The idea of this example is that here, as can be seen from the two-gray-squares picture, the density $g$ of $M$ is close to $0$ in a left neighborhood of $1/2$, which makes the monotonicity of $g$ impossible.
In this example, the pdf of $M$ is bimodal and has one (near-)zero in $(0,1)$. Similarly, for any $n\ge2$, by having $n$ non-overlapping "gray" squares cut in half by the diagonal of the unit square, we can make a saw-like pdf of $M$ with $n$ modes and $n-1$ (near-)zeroes in $(0,1)$.