Let $S$ be closed hyperbolic surface with genus $g\geq 2$. Let $Teich(S)$ be the Teichmueller space of $S$. It's well known that $Teich(S)$ is diffeomorphic to a (6g-6)-dimensional cell, where a coordinatization is given by the Fenchel-Nielsen length-twist coordinates $\{(\ell_a, \tau_a) \}_{a\in P}$ associated to a pant decomposition $P$ of $S$.

Question: can we replace the twist parameters with length parameters of other curves, and thereby replace the "length-twist" coordinates with "length-length" coordinates on $Teich(S)$?

Related question: are any formulas known which express the twist differentials $d\tau_a$ with linear combinations of length differentials $d\ell_{a'}$ in Wolpert's formula for the Weil-Petersson symplectic form $\omega_{WP}=\sum_{a\in P} d\ell_a \wedge d \tau_a$ ? I.e. can we express $\omega_{WP}$ using only differentials of length functions?

The same question: if we are given a hyperbolic surface $S'$ with known length parameters relative to a pant decomposition, then what metric properties on the surface $S'$ do we use to identify the twist parameters $\{\tau_a\}_a$?

Remark. This question risks being a duplicate. However we find the answer to the above question unsatisfactory, as indicated by our comments below.

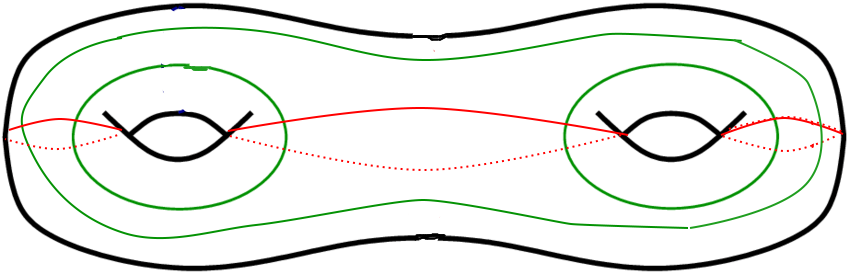
In genus $g=2$ we obtain the following almost canonical collection of six simple closed curves on the surface $S$. The lengths of the green curves do not distinguish between left and right Nielsen twists along the red curves.