I am studying enriched categories, and as I wrote in my previous question How is the morphism of composition in the enriched category of modules constructed?, this is very difficult because there are no elementary books on this topic (so I hope that specialists in category theory will not kill me for what I am asking here).
In particular, I am looking from time to time at the Tannaka duality theorem for modules over monoids in a symmetric monoidal category, and I have a feeling that it can be proved without the Yoneda lemma which is suggested as an instrument in this situation.
Am I right?
My impression is that everything must be simple, although I can't restore the details (and I hope that somebody will help me).
Suppose we have a monoid $A$ in a symmetric monoidal category $V$ and we consider the enriched category $_AV$ of modules over $A$, and the forgetful functor $F:{_AV}\to V$. It's easy to see that the family of maps $$ \alpha_X:A\to[F(X),F(X)], $$ where $\alpha_X$ corresponds to the $A$-module structure $\mu_X:A\otimes X\to X$ on $X\in\operatorname{Ob}({_AV})$, is what is called a wedge (from $A$ to $[F,F]$, or what is the correct term?).
Our aim is to prove that for any other wedge
$$
\beta_X:B\to[F(X),F(X)]
$$
there is a unique morphism $\omega:B\to A$ such that the diagrams in the family
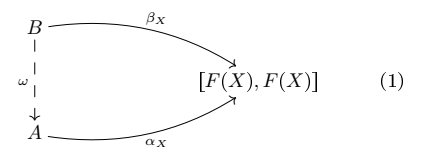
(with $X\in\operatorname{Ob}({_AV})$) are commutative.
I guess that this $\omega$ is defined as the composition $$ B\xrightarrow{\beta_A}[A,A] \xrightarrow{[\iota,1_A]}[I,A] \xrightarrow{\rho_{[\iota,1_A]}^{-1}}[I,A]\otimes I \xrightarrow{\operatorname{ev}^A_I}A $$ (where $I$ is the unit in $V$, $\iota:I\to A$ the unit in the monoid $A$ and $\rho_{[\iota,1_A]}$ the right identity for $[\iota,1_A]$), but I don't understand how to prove the commutativity of diagrams (1).
In particular, the special case of (1)
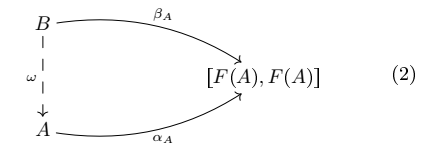
is also a puzzle for me. This is the same as the equality of the morphisms $$ B\otimes A\xrightarrow{\beta_A\otimes 1_A}[A,A]\otimes A \xrightarrow{[\iota,1_A]\otimes 1_A}[I,A]\otimes A \xrightarrow{\rho_{[\iota,1_A]}^{-1}\otimes 1_A}[I,A]\otimes I\otimes A \xrightarrow{\operatorname{ev}^A_I\otimes 1_A}A\otimes A \xrightarrow{\mu_A}A $$ and $$ B\otimes A\xrightarrow{\beta_A\otimes 1_A}[A,A]\otimes A \xrightarrow{\operatorname{ev}^A_A}A $$ but why are they equal? I can check this only in the situation when $A$ is a usual monoid (in the category of sets) or a usual algebra.
I would appreciate if anybody could show this "bookkeeping" (as Fosco Loregian calls it), because for an outsider like me this is incomprehensible. As I wrote in my previous question, each elementary step in this field is a problem, it requires weeks of pondering for me.
P.S. The applications of the Yoneda lemma are a gap in my education. If some kind person explains to me how it works, I will transfer this esoteric knowledge to the Moscow Mathematical School (with reference to this benefactor).
