The answer is no.
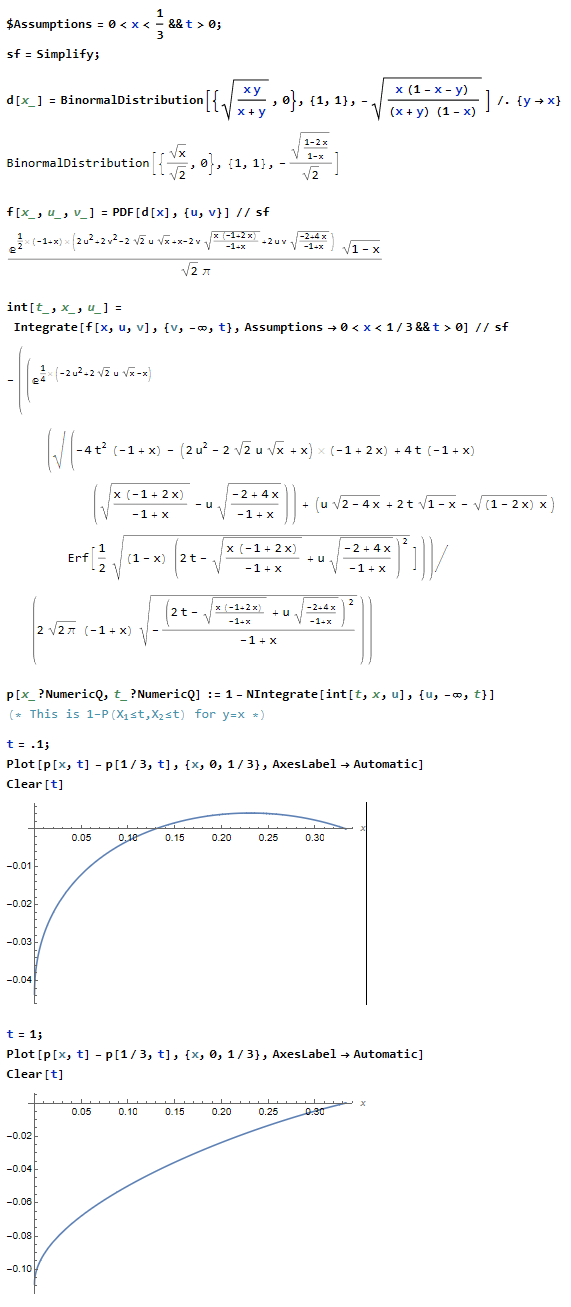
Let $x:=\xi_1$ and $y:=\xi_2$, so that $\xi_3=1-x-y$, $0\le x\le y\le1-x-y$, $$m:=\mu_1=\sqrt{\frac{x y}{x+y}},$$ $$r:=\rho=\sqrt{\frac{x(1-x-y)}{(x+y)(1-x)}}.$$ Let also $$p_t(x,y):=1-P(X_1\le t,X_2\le t).$$ We want to show that $$p_t(x,y)\le p_t(1/3,1/3) \tag{1}$$ for all $x,y$ as above and all real $t>0$.
However, inequality (1) fails to hold for small enough $t>0$ (say for $t=1/10$) if $x=y<1/3$ and $x$ is close enough to $1/3$.
Details are in a Mathematica notebook whose image is given below. (I can also prove the negative result rigorously, using a result by Plackett -- but is it really needed?)