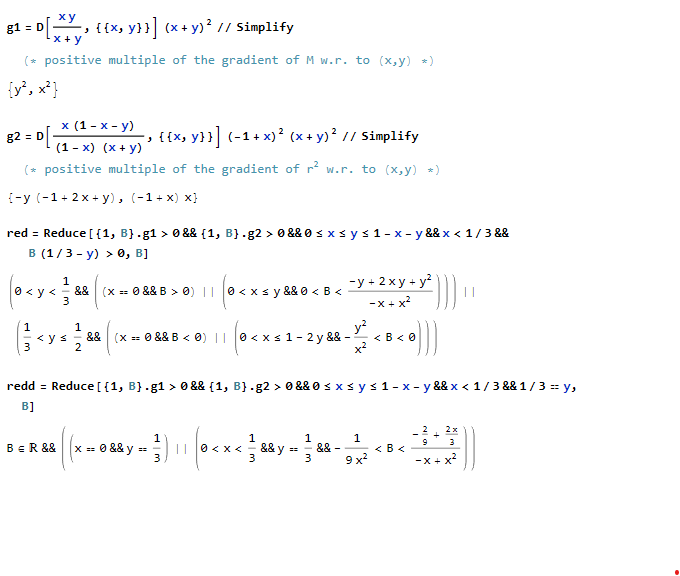Return to Answer

The answer is no.

Let $x:=\xi_1$ and $y:=\xi_2$, so that $\xi_3=1-x-y$, $0\le x\le y\le1-x-y$, $$m:=\mu_1=\sqrt{\frac{x y}{x+y}},$$ $$r:=\rho=\sqrt{\frac{x(1-x-y)}{(x+y)(1-x)}}.$$ Let also $$p_t(x,y):=1-P(X_1\le t,X_2\le t).$$ We want to show that $$p_t(x,y)\le p_t(1/3,1/3) \tag{1}$$ for all $x,y$ as above and all real $t>0$.

However, inequality (1) fails to hold for small enough $t>0$ (say for $t=1/10$) if $x=y<1/3$ and $x$ is close enough to $1/3$.

Details are in a Mathematica notebook whose image is given below. (I can also prove the negative result rigorously, using a result by Plackett -- but is it really needed?)

$\newcommand{\der}{\mathrm{der}}\newcommand{\tdert}{\mathrm{dert}}\newcommand{\erf}{\operatorname{erf}}\newcommand{\eqs}{\overset{\text{sign}}=}\newcommand{\tder}{\widetilde\der}$The answer is no.

Indeed, let $x:=\xi_1$ and $y:=\xi_2$, so that $\xi_3=1-x-y$, $0\le x\le y\le1-x-y$, whence $x\in[0,1/3]$.

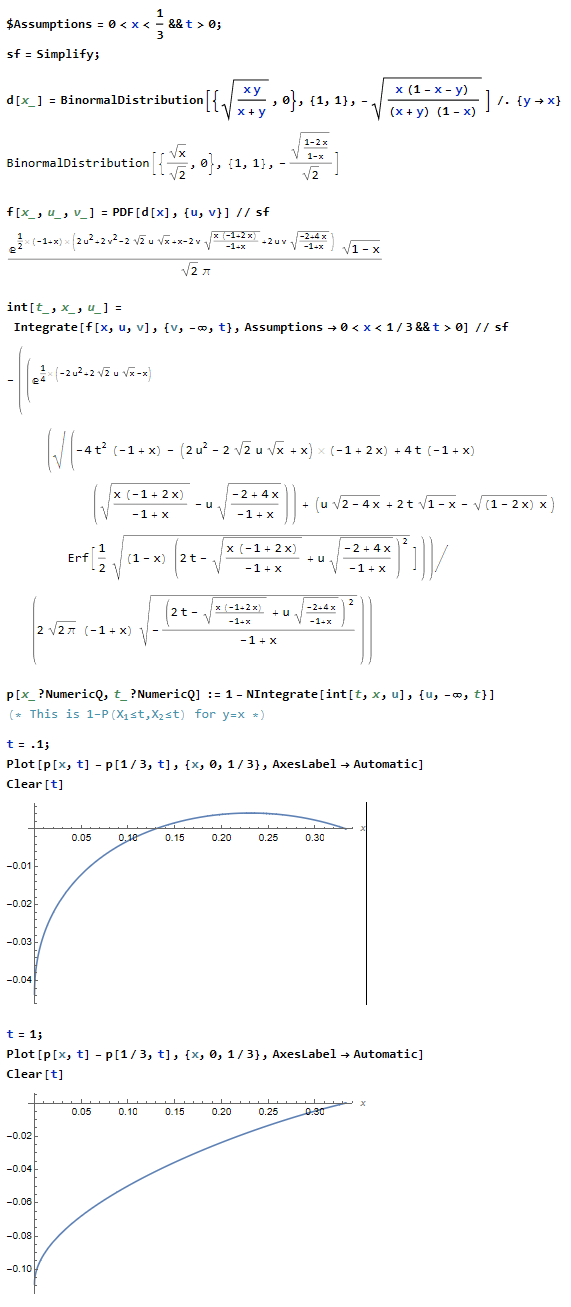
Consider further the case $y=x\in(0,1/3]$, so that $$\mu_1=M(x):=\sqrt{x/2},$$ $$\rho=-R(x),\quad R(x):=\sqrt{\frac{1-2x}{2(1-x)}}.$$ Let also $$p_t(x):=1-P(X_1\le t,X_2\le t).$$ Your desired result would imply that \begin{equation*} p_t(x)\le p_t(1/3) \tag{0} \end{equation*} for all $x\in(0,1/3]$ and all real $t>0$.

Note that \begin{equation*} p_t(x)=1-P(X_1\le t,X_2\le t)=P(M(x),-R(x)), \tag{1} \end{equation*} where \begin{equation*} P(m,r):=1-\int_{-\infty}^t du \int_{-\infty}^t dv\, f_{m,r}(u,v) \end{equation*} and $f_{m,r}$ is the density function of the bivariate normal distribution with means $m,0$, variances $1,1$, and correlation $r$.

The key is Plackett's observation (formula (3)) that \begin{equation*} D_r f_{m,r}(u,v)=D_v D_u f_{m,r}(u,v), \end{equation*} where $D_w$ denote the partial derivative with respect to a variable $w$. It follows that \begin{equation*} \begin{aligned} D_r P(m,r)&:=-\int_{-\infty}^t du \int_{-\infty}^t dv \, D_r f_{m,r}(u,v) \\ &=-\int_{-\infty}^t du \int_{-\infty}^t dv \, D_v D_u f_{m,r}(u,v) \\ &=-f_{m,r}(t,t). \end{aligned} \tag{2} \end{equation*} Next, \begin{equation*} \begin{aligned} P(m,r)&=1-\int_{-\infty}^t du \int_{-\infty}^t dv\, f_{0,r}(u-m,v) \\ &=1-\int_{-\infty}^{t-m} dw \int_{-\infty}^t dv\, f_{0,r}(w,v) \end{aligned} \end{equation*} and hence \begin{equation*} \begin{aligned} D_m P(m,r)=\int_{-\infty}^t dv\, f_{0,r}(t-m,v)=\int_{-\infty}^t dv\, f_{m,r}(t,v); \end{aligned} \tag{3} \end{equation*} the latter integral can be easily expressed in terms of the error function $\erf$ and elementary functions.

By (1) and a chain rule of differentiation, \begin{equation*} D_x p_t(x)=D_m P(M(x),-R(x))M'(x)-D_r P(M(x),-R(x))R'(x). \end{equation*}

In particular, \begin{equation} D_x p_{1/10}(x)\big|_{x=1/3}=\erf\left(\frac{1}{60} \left(3 \sqrt{6}-10\right)\right)+1-\frac{3 }{\sqrt{\pi }}\,e^{(30 \sqrt{6}-77)/1800} =-0.739\ldots<0. \end{equation} So, inequality (0) fails to hold for $t=1/10$ and all $x$ in a left neighborhood of $1/3$. $\quad\Box$

The answer is no.

Let $x:=\xi_1$ and $y:=\xi_2$, so that $\xi_3=1-x-y$, $0\le x\le y\le1-x-y$, $$m:=\mu_1=\sqrt{\frac{x y}{x+y}},$$ $$r:=\rho=\sqrt{\frac{x(1-x-y)}{(x+y)(1-x)}}.$$ Let also $$p_t(x,y):=1-P(X_1\le t,X_2\le t).$$ We want to show that $$p_t(x,y)\le p_t(1/3,1/3) \tag{1}$$ for all $x,y$ as above and all real $t>0$.

However, inequality (1) fails to hold for small enough $t>0$ (say for $t=1/10$) if $x=y<1/3$ and $x$ is close enough to $1/3$.

Details are in a Mathematica notebook whose image is given below:

The answer is no.

Let $x:=\xi_1$ and $y:=\xi_2$, so that $\xi_3=1-x-y$, $0\le x\le y\le1-x-y$, $$m:=\mu_1=\sqrt{\frac{x y}{x+y}},$$ $$r:=\rho=\sqrt{\frac{x(1-x-y)}{(x+y)(1-x)}}.$$ Let also $$p_t(x,y):=1-P(X_1\le t,X_2\le t).$$ We want to show that $$p_t(x,y)\le p_t(1/3,1/3) \tag{1}$$ for all $x,y$ as above and all real $t>0$.

However, inequality (1) fails to hold for small enough $t>0$ (say for $t=1/10$) if $x=y<1/3$ and $x$ is close enough to $1/3$.

Details are in a Mathematica notebook whose image is given below. (I can also prove the negative result rigorously, using a result by Plackett -- but is it really needed?)

Let $x:=\xi_1$ and $y:=\xi_1$, so that $\xi_3=1-x-y$, $0\le x\le y\le1-x-y$, $$m:=\mu_1=\sqrt{\frac{x y}{x+y}},$$ $$r:=\rho=\sqrt{\frac{x(1-x-y)}{(x+y)(1-x)}}.$$ Let also $$p_t(x,y):=P_t(m,r):=1-P(X_1\le t,X_2\le t).$$

Note that $P_t(m,r)$ is increasing in $m$, because an increase of $m$ by $h$ can be modeled by replacing $X_1$ by $X_1+h$.

Also, by Slepian's lemma, $P_t(m,r)$ is increasing in $r$.

Fix any real $t$. Let now $(x,y)$ be a point of maximum of $p_t(x,y)$ over all $(x,y)$ subject to the conditions $0\le x\le y\le1-x-y$. These conditions imply $0\le x\le1/3$. Moreover, if $x=1/3$, the conditions $0\le x\le y\le1-x-y$ imply $y=1/3$, and we are done.

Finally, if $x\ne1/3$ (that is, if $x<1/3$), then there always exists a vector $v$ of the form $(1,B)$ for a real $B$ such that the directional derivatives of both $m$ and $r$ in the direction of $v$ are $>0$, and small enough movements in this direction preserve the conditions $0\le x\le y\le1-x-y$. See details of these calculations with Mathematica in the image below (click on the image to enlarge it). So, such a movement will increase the value of $p_t$, which contradicts the assumption that $(x,y)$ is a point of maximum.

Thus, the only point of maximum is $(x,y)=(1/3,1/3)$, as desired.

The answer is no.

Let $x:=\xi_1$ and $y:=\xi_2$, so that $\xi_3=1-x-y$, $0\le x\le y\le1-x-y$, $$m:=\mu_1=\sqrt{\frac{x y}{x+y}},$$ $$r:=\rho=\sqrt{\frac{x(1-x-y)}{(x+y)(1-x)}}.$$ Let also $$p_t(x,y):=1-P(X_1\le t,X_2\le t).$$ We want to show that $$p_t(x,y)\le p_t(1/3,1/3) \tag{1}$$ for all $x,y$ as above and all real $t>0$.

However, inequality (1) fails to hold for small enough $t>0$ (say for $t=1/10$) if $x=y<1/3$ and $x$ is close enough to $1/3$.

Details are in a Mathematica notebook whose image is given below: