Let $p_i = [x_i,y_i]$ be the vertices of the $n$-gon. To minimize the $m$-gon covering it, we minimize $r$ subject to the constraints
$$ \cos(\theta + 2 \pi j/m) (x_i - x) + \sin(\theta+2\pi j/m) (y_i - y) \le r $$ with respect to the variables $\theta, x, y, r$. Here $(x,y)$ represents the coordinates of the centre of the $m$-gon.
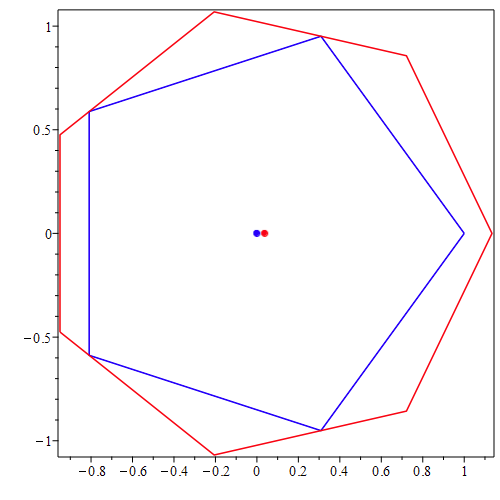
In the case $n=5$, $m=7$, with $x_i = \cos(2 \pi i/5)$, $y_i = \sin(2\pi i/5)$, using numerical methods in Maple I find the minimum is approximately $r = 0.987554526096907$ for $\theta = \pi$, $x = 0.0378380934711730$, $y = 0$. On the other hand, the concentric case would have $x=0$, $y=0$, and a minimum $r$ of approximately $0.995974293995239$, which is significantly greater, again for $\theta=\pi$.
EDIT: Here is a picture of the optimal solution. The centre of the pentagon is in blue, the centre of the heptagon in red.