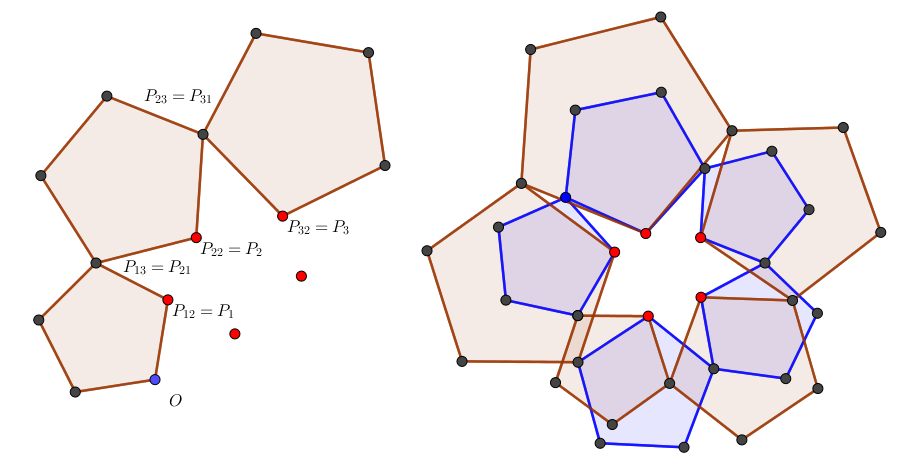
I posed a generalization of Theorem 3.2 In my paper

Conjecture: Let $P_1, P_2,....,P_{2n+1}$ and $O$ be $2n+2$ points in plane. Construct a chain $2n+1$ regular ${2n+1}$-gons $P_{11}P_{12}...P_{1\;2n+1}$; ....;$P_{2n+1\;1}P_{2n+1\;2}...P_{2n+1\;2n+1}$ such that $O=P_{11}$, $P_{12}=P_1$, $P_{2\;1}=P_{13}$, $P_{2\;2}=P_{2}$, $P_{i+1\;1}=P_{i3}$, $P_{i+1\;2}=P_{i+1}$ for $i=\overline{1\;2n}$

Continuing construct a chain $2n+1$ regular ${2n+1}$-gons $A_{11}A_{12}...A_{1\;2n+1}$; ....;$A_{2n+1\;1}A_{2n+1\;2}...A_{2n+1\;2n+1}$ such that $A_{11}=P_{2n+1\;3}$, $A_{12}=P_1$, $A_{2\;1}=A_{13}$, $A_{2\;2}=P_{2}$, $A_{i+1\;1}=A_{i3}$, $A_{i+1\;2}=P_{i+1}$ for $i=\overline{1\;2n}$.

Then $A_{2n+1\;3}=O$

Corollary: $A_{2n+1\;3}$ is fixed point when $P_1$, $P_2$, ....,$P_n$ be moved.

Question 1: Is the conjecture correct?

Question 2: Let $P_1$, $P_2$, ....,$P_n$ are fixed point in the plane, find position of $O$ such that $P_{i1}P_{i2}...P_{i\;2n+1}=A_{i1}A_{i2}...A_{i\;2n+1}$ for $i=\overline{1\;2n+1}$

PS: In the conjecture, all regular polygon is same direction.