Recall that an open cover $\mathfrak{U} = \{ U_\alpha \}$ of a manifold $M$ is called a good cover if all possible finite intersections $U_{\alpha_1} \cap ... U_{\alpha_n}$ are contractible.

Question: What is the minimum number of open sets required for a good cover of the 2-dimensional torus?

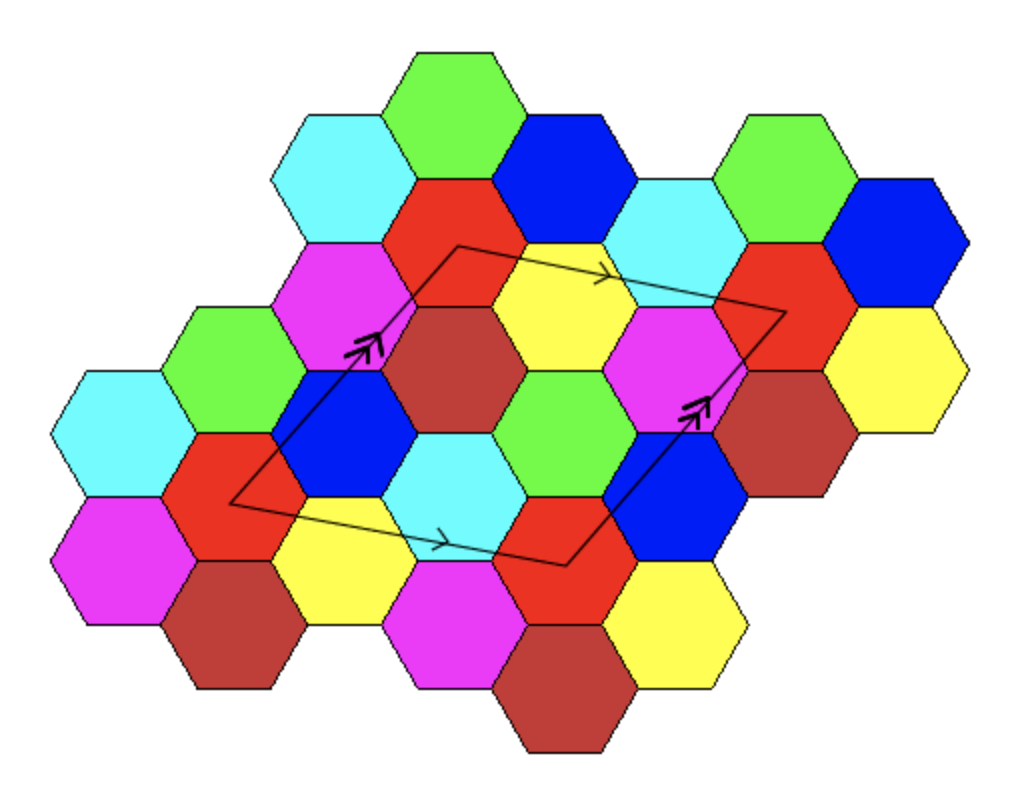
The picture below provides a good cover of the torus (i.e. opposite sides of the parallelogram identified as usual) using 7 open sets (i.e. take sufficiently small open neighbourhoods of the hexagons). Can one do any better than 7? If not, how does one prove that 7 is optimal?