I was inspired by this topic on Math.SE.
Suppose that $H_n = \sum\limits_{k=1}^n \frac{1}{k}$ - $n$th harmonic number. Then

Conjecture

Let $M$ be a set of all $n$ such that $$H_n - \lfloor{H_n\rfloor} < \frac{1}{n^{1+\epsilon}}.$$ Then $$\forall\epsilon>0 : |M| = \bar\eta(\epsilon) < \infty.$$

Picture below illustrates my conjecture, where I have checked this conjecture for $n < 10^6$ for each $\epsilon\in(0,1.1)$ with step 0.01

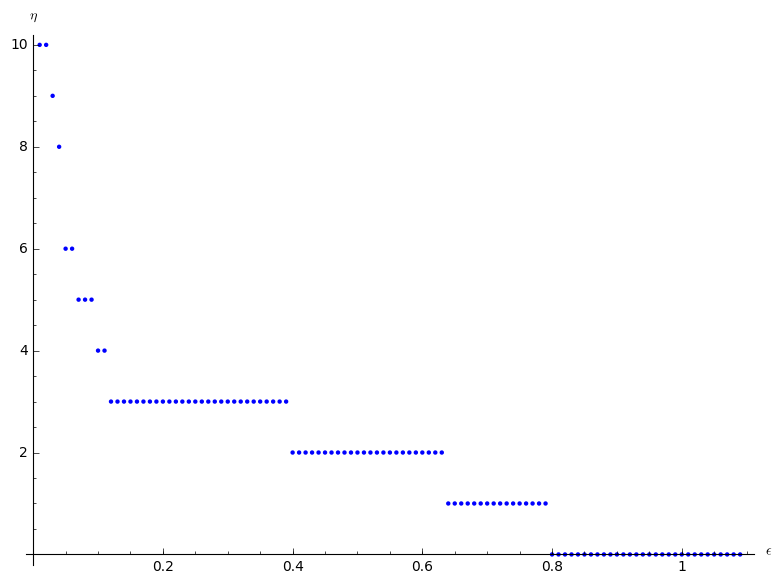
Please, let me know if it is obviously (or not obviously) false.