As the title suggests, I'm trying to apply the Adams Spectral sequence to get some insights of the bordism group $$ \Omega_4(\xi)= \pi_4(M\xi)$$ where $\xi \colon BSpin \times K(D_{2n},1) \to BSO$ is a stable vector bundle. I'm trying to use ASS because after an application of the James Spectral Sequence (kind of twisted AHSS) I was able to conclude that $$ \Omega_4(\xi)= \mathbb{Z} \ \text{ or } \mathbb{Z}\oplus \mathbb{Z}_2$$ Here you can find a little bit of context and some description of my previous attempts.

My idea is that a computation of $_{(2)}{\pi_4(M\xi)}$ should give me the right choice for $\Omega_4(\xi)$, therefore I try to run an ASS. My lack of expertise in this field lead me to ask a question about how to start the ASS, since I think it's easier to study the ASS with a clear problem in mind, otherwise I wouldn't understand the importance of a lot of technical lemmas done in many of the books covering it.

So the ASS I'm interested in should look like this $$E^{s,t}_2\cong Ext_{\mathcal{A}_2}^{s,t}(H^*(M\xi;\mathbb{Z}_2); \mathbb{Z}_2)$$

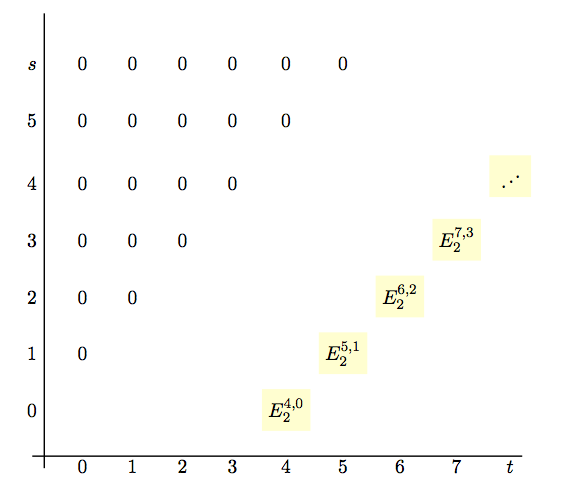
where the yellow diagonal is the one I'm interested in. A first glance to it lead me to this question:

(1) How can I conclude something if the diagonal contains infinitely many non-zero stable terms?

Even computing the $2$-page is troublesome. According to what I know (I've read the chapter about ASS in Fomenko-Fuchs Homotopical Topology), I should find a (minimal)-projective resolution of the the $\mathcal{A}_2$-module $H^*(M\xi;\mathbb{Z}_2)$ which via Thom iso I think can be seen as $H^*(BSpin ;\mathbb{Z}_2)\otimes H^*(D_{2n};\mathbb{Z}_2)$. Problem is that I'm supposed to find an infinitely long (minimal) resolution $B_{\bullet}\to H^*(M\xi)$, since for example $E^{s,t}_2=\hom_{\mathcal{A}_2}(B_s,\mathbb{Z}_2)$ so I really need to compute $B_s$ for all $s$ (and for every internal grade $t$).

(2) How can (cleverly) I compute at least the second page of this ASS?

The reason I'm asking these questions is that (as you can see in the linked question) I was suggested to use ASS, so I believe something could be said in this case and I'm really interested in learning how to use this powerful tool. The big amount of algebra (at least for a non-algebrist grad student like me) involved in the ASS is making difficult to me getting used to such a tool.

I'm aware that there are plenty of material about the ASS in the books and online, but I prefer learning them by getting my hands dirty with concrete examples I'm really interested in computing, otherwise I fear I will get lost in the ocean of literature about ASS