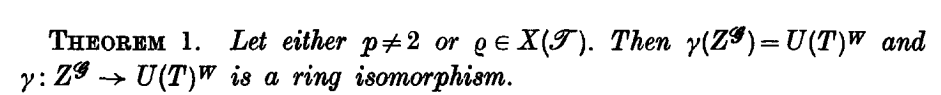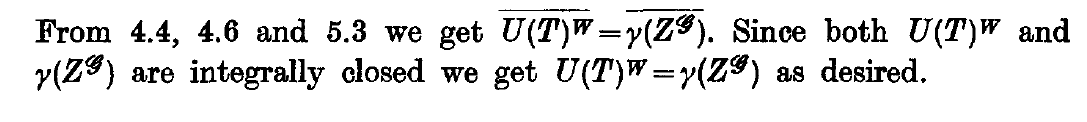I am trying to understand the proof of theorem 1 from this paper V. Kac and B. Weisfeiler.

Here $Z$ is the center of universal enveloping algebra $U(G)$ (here $G = \operatorname{Lie} \mathscr{G}$ ). $T$ is a Lie algebra of a maximal torus. $Z^{ \mathscr{G} }$ denote invariants (with respect to Ad-action of group $\mathscr{G}$) in $Z$.

Proof is not very short. But the last two lines are these

Here $\bar{A}$ means field of fraction of ring $A$. Suppose I believe that these fields are isomorphic and these rings are integrally closed.

Question How does it imply isomorphism of initial rings?

For example $ \mathbb{k} [x, xy] \subset \mathbb{k} [x, y]$