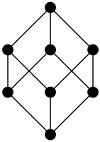
The rank $n$ boolean lattice $B_{n}$ is the subset lattice of $\{1,2, \dots , n\}$.
The lattice $B_{3}$ is the following:

Question: What are the rank $3$ boolean intervals of the form $[H,G]$, with $G$ a simple group?

Remark: For $\vert G \vert \leq 4000000$, we have found (by GAP):

• $A_8$ (of order $20160$) with a subgroup of index $315$,
• $PSU(3,5)$ (of order $126000$) with a subgroup of index $6000$,
• $PSp(6,2)$ (of order $1451520$) with a subgroup of index $2835$,
• $PSU(4,3)$ (of order $3265920$) with a subgroup of index $25515$.

Can we have a classification in general?