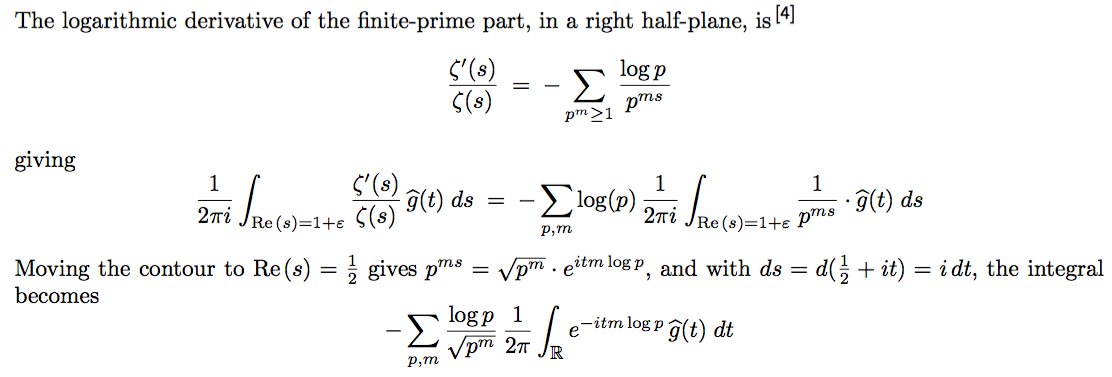This might be a silly question.

In all proofs of the Weil-Guinand explicit formula, there's a step similar to this (this is from Paul Garrett's notes):

Now consider this: $\frac{\zeta^\prime(s)}{\zeta(s)}$ has poles at $s=1$ and $s=\frac{1}{2} + i \gamma$, so if we move the contour from $\Re(s) = 1+\epsilon$, to $\Re(s) = \frac{1}{2}$, we'll pick up all the residues.

Yet if we write out the Dirichlet series of $\frac{\zeta^\prime(s)}{\zeta(s)}$, then $p^{-ms}$ has no pole between $\Re(s) = 1+\epsilon$ and $\Re(s) = \frac{1}{2}$, so the proof claim we can move the contour to $\Re(s) = \frac{1}{2}$ without picking up any residues. Is this really justified?