The free subgroups consist of the closure of the Riley slice of Schottky space.
Here's a picture:
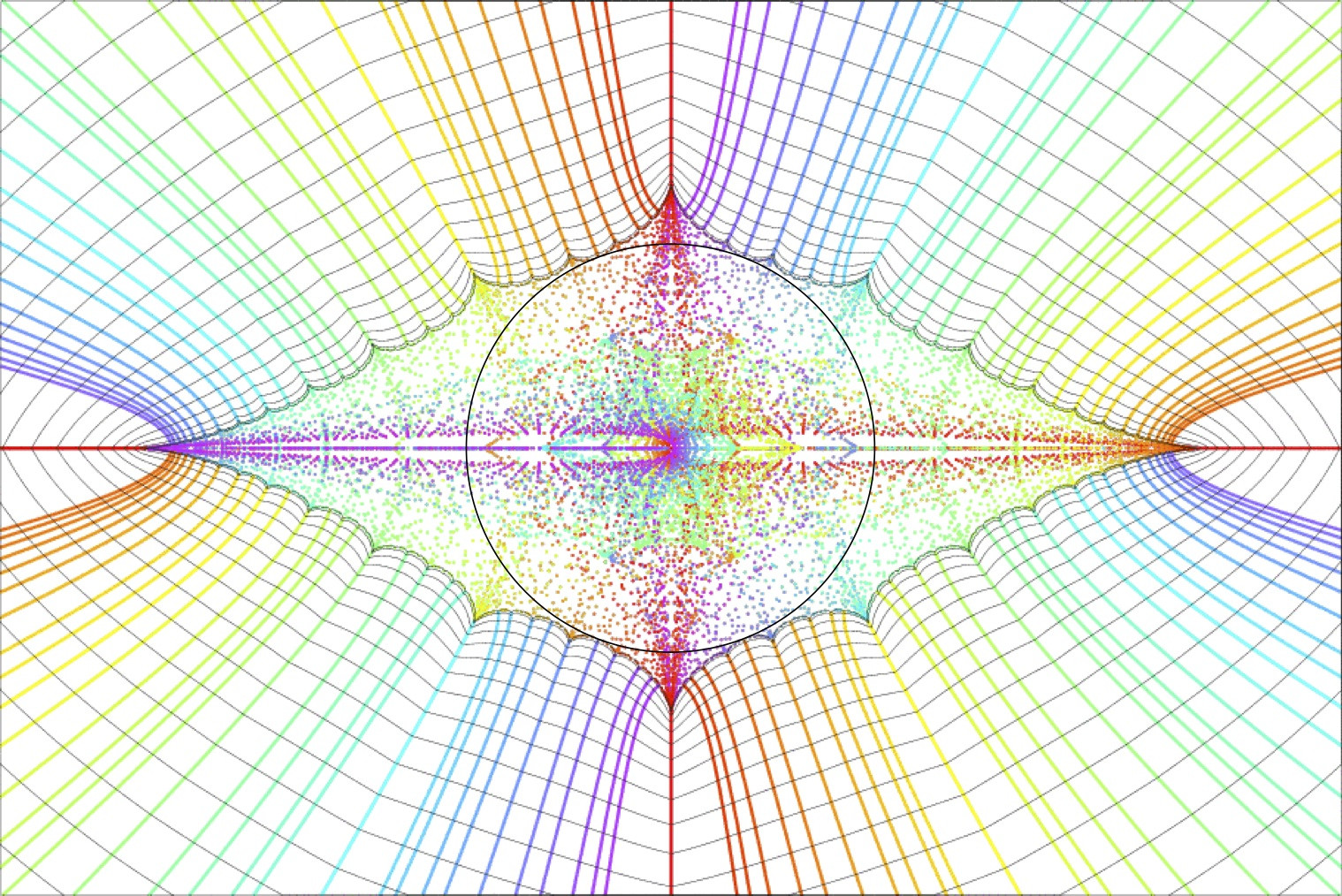
The exterior of the black fractal represents free discrete groups that are generalized Schottky groups. The black curve consists of geometrically finite groups with a cusp, or degenerate groups. By the density conjecture, it is known that all free two-parabolic generator groups lie in the boundary of the Riley slice.
In the interior, there are many more non-free discrete groups, such as those corresponding to 2-bridge links. I'll add some references on these topics later. See also some incomplete notes of mine.
