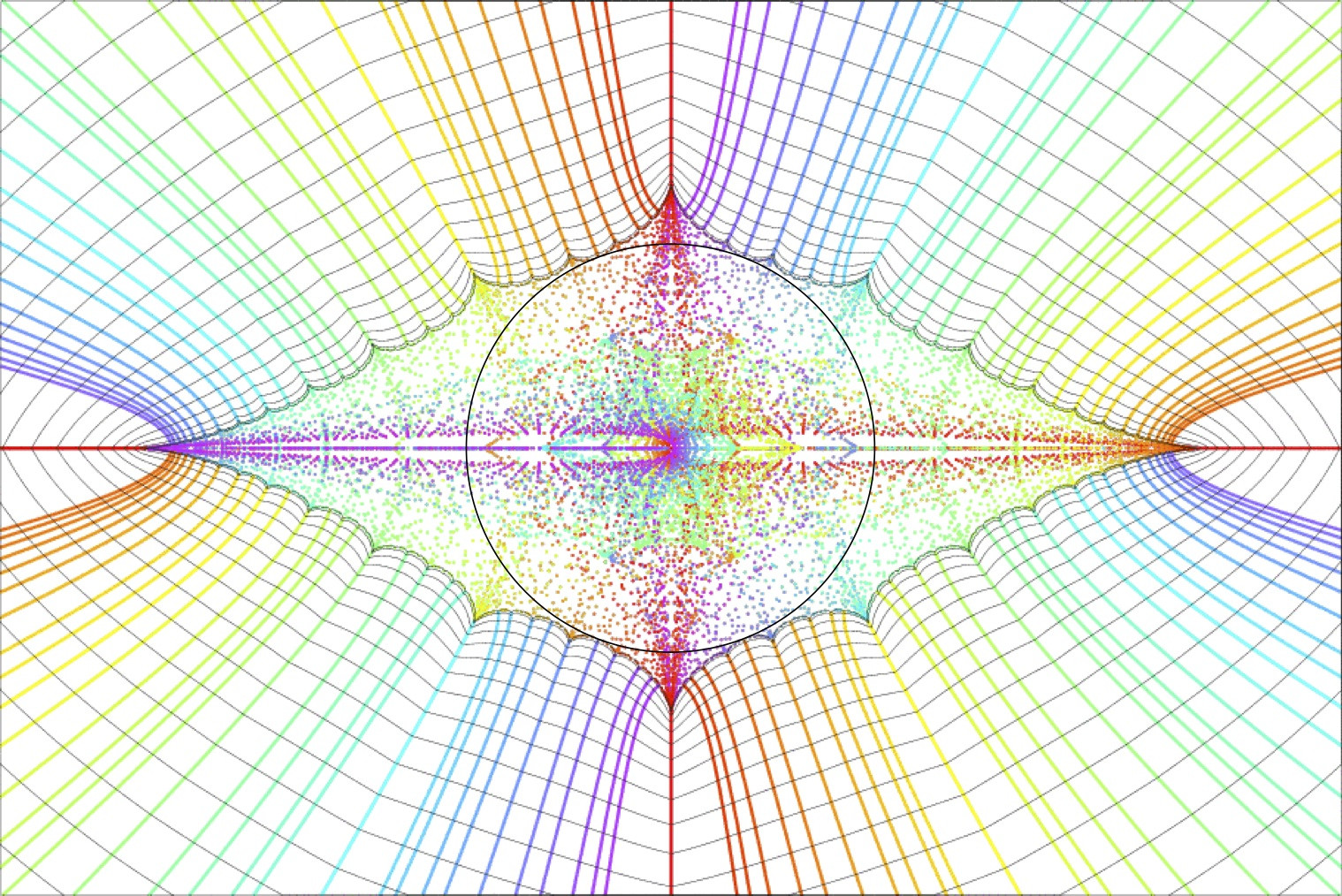
The free discrete subgroups consist of the closure of the Riley slice of Schottky space (notice that one may assume $Re(\alpha)\geq 0, Im(\alpha)\geq 0$, since $\alpha \mapsto -\alpha, \overline{\alpha}$ preserves discreteness).
Here's a picture of the first quadrant of the Riley slice:
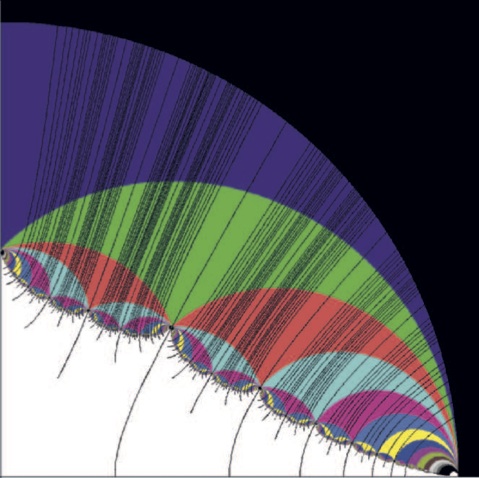
The exterior of the black fractal represents free discrete groups that are generalized Schottky groups. The colored regions correspond to the combinatorics of the Ford domain. These have been investigated in detail by Akiyoshi, Sakuma, Wada, and Yamashita (see also their monograph where the picture was taken from). The black curve consists of geometrically finite groups with a cusp or degenerate groups. By the density conjecture, it is known that all free two-parabolic generator groups lie in the boundary of the Riley slice. Moreover, it is known that there is a group realizing each ending lamination parameterized by $\mathbb{R/Z}-\mathbb{Q/Z}$.
In the exterior of the Riley slice, there are many more non-free discrete groups, such as those corresponding to 2-bridge links. In fact, all of the torsion-free discrete groups correspond to 2-bridge links. Note that for 2-bridge links, although they are known to be generated by 2 parabolics, the precise value of $\alpha$ which gives the parabolics has not been determined. One may see an example of this for the twist knots, for which $\alpha$ has been worked out by Hoste and Shanahan.
For the discrete groups with torsion, there are certain other orbifolds closely related to the 2-bridge links; see also some incomplete notes of mine. Conjecturally, all of the discrete groups are obtained by extending the "pleating locus" for each rational number $\in \mathbb{Q/Z}$ (representing a simple closed curve on the 4-punctured torus) through the cusp, and into the complement of Schottky space. The points where these elements are primitive elliptics should correspond to discrete groups (with some caveats); these pleating rays are displayed in the picture. In fact, the discrete groups in the complement of the Riley slice closure form a discrete set, with limit points at the boundary of the Riley slice.