I posted the following question on math stackexchange but I have not received any answer. So I hope people here can help me.

In the book, Lectures on tensor categories and modular functors by Bakalov and Kirillov they construct a TQFT.

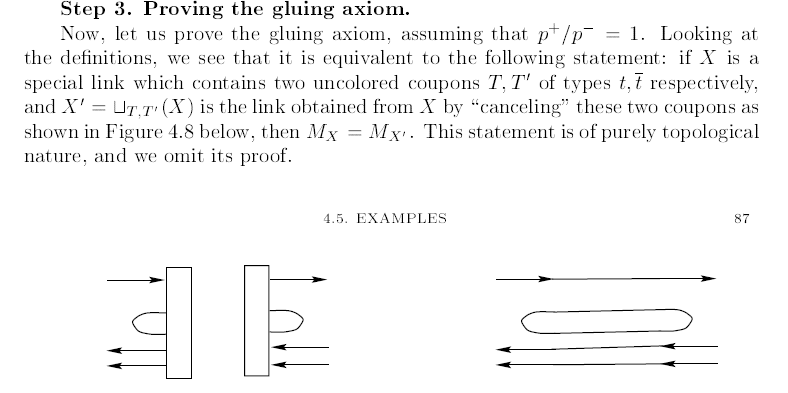
When they come to prove the gluing axiom, they just mention that "...This statement is of purely topological nature, and we omit its proof." And I don't see how to prove it.

Basically, they claim that if we cancel two coupons of a special link we get the same manifold via surgery. (See the picture from page 87 of the book)

Could someone provide me a proof of the gluing axiom or give me a reference containing a complete proof? I read a Turaev's book but that proof is also not easy to understand.