Shadow boundary on convex body in $\mathbb{R}^3$

Question

Let $S$ be the surface of a compact, convex, smooth ($C^\infty$) body in $\mathbb{R}^3$, with strictly positive Gaussian curvature at every point of $S$. Fix a direction $z$ in a Cartesian coordinate system, and consider all the lines parallel to $z$ and tangent to $S$, which form a topological cylinder enclosing $S$, touching $S$ on the shadow boundary resulting from a light source at $z=+\infty$ (yellow in the figure below). Parametrize these lines from $s=0$ to $s=1$ around the cylinder, and let $h(s)$ be the height of the point of tangency to $S$ above the $xy$-plane, orthogonal to $z$. My question is:

Can $h(s)$ have an arbitrarily large number of local maxima and minima?

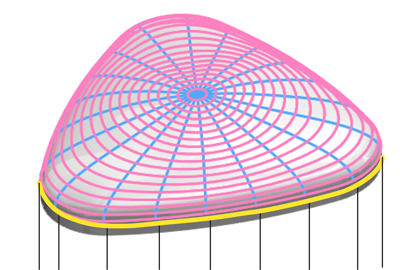
I am interested to learn if this shadow-boundary curve is "well-behaved" in some sense, for smooth convex bodies. Thanks for pointers/suggestions/counterexmaples!

Answer 1

The shadow boundary can be any $C^\infty$ curve with (quadratically) strictly convex projection to the $xy$-plane. For simplicity, let me stick to the case when the projection is a circle.

So consider a smooth function $h:S^1\to\mathbb R$. I am going to construct a smooth, compact, quadratically convex surface whose shadow boundary equals the set $\{(s,h(s)):s\in S^1\}\subset S^1\times\mathbb R\subset\mathbb R^2\times\mathbb R$. Here $S^1$ is the standard circle $x^2+y^2=1$ in the $xy$-plane.

For an $\varepsilon>0$, define a function $F_\varepsilon:\mathbb R^3\to\mathbb R$ by $$ F_\varepsilon(x,y,z) = x^2+y^2+z^2(1-\varepsilon h(\theta(x,y))) $$ where $\theta(x,y)\in S^1$ is the angular coordinate of the point $(x,y)$ in the plane: $\theta(x,y)=(x,y)/\sqrt{x^2+y^2}$. Let $F_\varepsilon$ be the set of solutions of the equation $F_\varepsilon(x,y,z)=1$.

As $\varepsilon\to 0$, the function $F_\varepsilon$ converges to $F_0$ in $C^\infty$ (in a neighborhood of the sphere $x^2+y^2+z^2=1$), where $F_0(x,y,z)=x^2+y^2+z^2$. Since the limit equation $x^2+y^2+z^2=1$ is non-degenerate (in the sence of the implicit function theorem), so is the equation $F_\varepsilon(x,y,z)=1$ for a sufficiently small $\varepsilon$. Hence $S_\varepsilon$ is a smooth surface close to the sphere. Furthermore, its first and second derivatives are close to those of the sphere, therefore $S_\varepsilon$ has positive second fundamental form and hence is convex.

The shadow boundary of $S_\varepsilon$ is the set of points $(s,\varepsilon h(s)):s\in S^1$. It remains to apply the affine transformation $(x,y,z)\mapsto (x,y,z/\varepsilon)$ to get the original height function $h$.

Answer 2

Here's a way to think about it. Suppose you have a curve in space that projects to a convex curve in the plane with nonvanishing cuvature at each point. Think of making a slight modification of the cylinder with given projection: turn a finite portion of it into a barrel, replacing each generating line by an arc of a circle with very large radius whose tangent where it intersects the curve is vertical and whose center is on the horizontal ray perpendicular to the curve and pointing inward.

If the curvature of the circles is small enough, then the sides of the barrel are convex. That's because the vertical slices are convex, the horizontal slices are convex; shifting the circles up and down adds to a cross term in the second fundamental form, tending to "warp" the surface, but as long as the circles are nearly vertical compared to the vertical motion, this cross term is small enough that the surface remains vertical.

The sides of the barrel can easily be capped at the top and bottom to make a smooth, strictly convex surface.

Answer 3

This is not an answer but a shadow tangent Mohammad Ghomi has a wonderful paper concerning a converse question: what are neccessary and sufficient conditions on the shadows which insure the surface to be convex. His answer: for all projection directions, the shadow must be connected and simply connected. See: Solution to the shadow problem in 3-space, in Minimal Surfaces, Geometric Analysis and Symplectic Geometry, Adv. Stud. Pure Math, 34 (2002) 129-142. Which you can find on his web page.