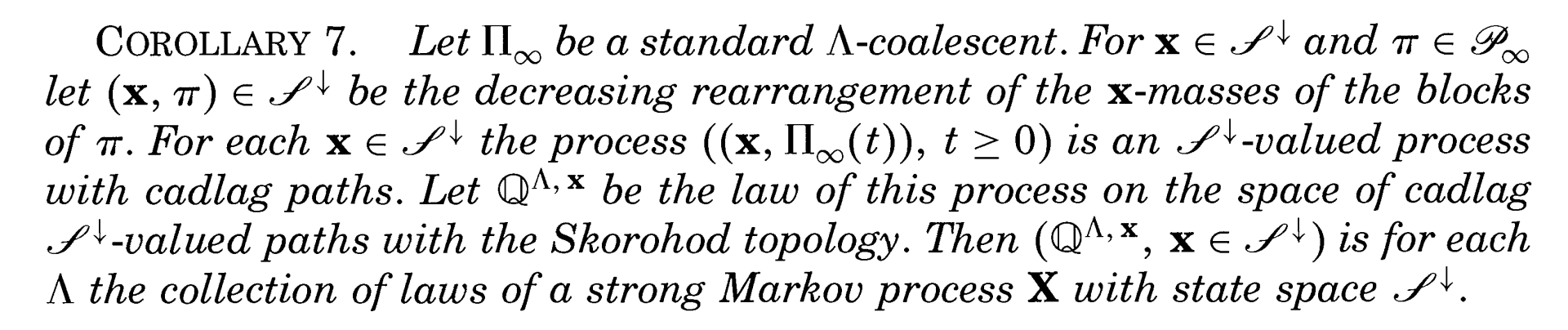
I am reading a paper by Pitman (1999), and I am confused by his Corollary 7. First some notation so that I can explain my confusion:
- $\mathcal{P}_\infty$ is the space of partitions of $\mathbb{N}$, $\mathcal{P}_n$ is its restriction to $[n] = \{1, 2, \ldots, n\}$.
- $\Pi_\infty^\pi(t)$ is a $\Lambda$-coalescent on $\mathcal{P}_\infty$ with $\Pi_\infty^\pi(0) = \pi \in \mathcal{P}_\infty$ and $\Pi_n^\pi(t)$ is its restriction to $\mathcal{P}_n$. A standard $\Lambda$-coalescent is one that starts at $\pi = \{\{1\},\{2\},\ldots\}$.
- $\mathcal{S}^\downarrow = \{x \in \ell^1: x_1 \geq x_2 \geq \ldots, \, \sum x_i = 1\}$. In other words, $\mathcal{S}^{\downarrow}$ is the set of ranked probability distributions on $\mathbb{N}$.
I am confused about the second sentece in the corollary. Clearly, there is a ranked rearrangement of the masses of $\pi$, but what is meant by "The $\mathbf{x}$-masses of $\pi$"? What I understand is that $\mathbf{x}$ is a particular element of $\mathcal{S}^\downarrow$ and $\pi$ is a particular element of $\mathcal{P}_\infty$ whose ranked mass rearrangement may or may not coincide with $\mathbf{x}$, hence my confusion.
Could someone explain to me what is meant by the $\mathbf{x}$-masses of $\pi$ and what the process $((\mathbf{x}, \Pi_\infty(t)), t\geq 0)$ looks like?