In chapter 6 of HA by Lurie, for any functor $F:C\rightarrow D$ between 'nice' categories (like differentiable infinity categories), there is an $n$ excisive approximation to this functor behaving like the Taylor expansion. For the 1 excisive case, there is a formula given by Example 6.1.1.23 and Example 6.1.1.28 in HA: \begin{equation} P_{1}F=\varinjlim_{k} \Omega^{k}_{D}\circ F\circ \Sigma^{k}_{C} \end{equation}
$\Sigma_{C}(X)$ is suspension functor defined as the homotopy cofiber of $X\rightarrow *$, and $\Omega_{D}$ is the loop functor in $D$ defined as the homotopy fiber of the pointed object $*\rightarrow Y$, here $\Sigma X$ is automatically a pointed object by definition, so $\Omega_{D}^{k}\circ F\circ \Sigma^{k}$ makes sense.
For the identity functor between the category of pointed topological spaces $Spc_{*}$, $\varinjlim_{k}\Omega^{k}\Sigma^{k}(X)$ is exactly the 'stablization' of topological space $X$, that is, the 0th component of the spectrification of the sequential spectrum $\{X\wedge S^{k}\}_{k}$, so it's justifiable to write the 1 excisive approximation to the identity functor as $\Omega^{\infty}\Sigma^{\infty}(X)$ where $\Sigma^{\infty}$ is the infinite suspension functor from $Spc_{*}$ to $Sp(Spc_{*})$, which is the left adjoint to the infinite loop functor $\Omega^{\infty}:Sp(Spc_{*})\rightarrow Spc_{*}$.
But for the general case of an identity functor $id_{C}:C\rightarrow C$ with $C$ a differential infinity category, for instance $E_{\infty}$ ring over $B$: $C=CAlg_{/B}$, I don't know about the relationship between the infinite suspension $\Sigma^{\infty}:C\rightarrow Sp(C)$ and the suspension functor $\Sigma: C\rightarrow C$, and also the 1 excisive approximation is unclear. What I do know is the derivative $\partial id_{C}$ is still the identity functor $id_{Sp(C)}:Sp(C)\rightarrow Sp(C)$ by Example 6.1.2.4 in HA, the natural isomorphism $id_{C}\circ \Omega^{\infty}=\Omega^{\infty}\circ id_{Sp(C)}$ exhibits identity functor as derivative of identity functor.
Intuitively, there should be some $\textbf{relationship}$ between this 'first derivative' and the 1 excisive approximation, for the case when $C=Spc_{*}$, there are many results on this topic, but I am wondering
$\textbf{Question:}$ $\textbf{What happens for the general case when $C$ is an unpointed differentiable category}$, $\textbf{like}$ $\mathbf{CAlg_{/B}}$?
$\textbf{Remark 1:}$
I guess there must be some materials explaining this general case, because as I can see, in definition 2.2.3 of this paper, where $I$ adic completion $\widehat{R}$ is defined through GoodWillie calculus. And moreover, it seems that for $A\in CAlg_{B}$, the 1 excisive approximation of identity functor is exactly the universal square zero extension $P_{1}F(A\rightarrow B)=fib(P_{0}F(A)=B\stackrel{d}{\longrightarrow}B\oplus L_{B/A})$ where $d$ is the universal derivation corresponding to $L_{B/A}\stackrel{id}{\longrightarrow} L_{B/A}$,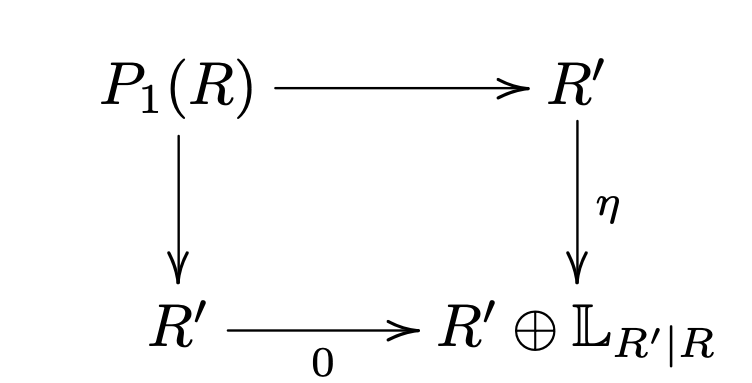
$\textbf{Observation:}$
I do know that the relative cotangent $L_{B/A}$ is the cofiber of $\{\Sigma^{\infty}(A\rightarrow B)=f_{*}L_{A} \rightarrow \Sigma^{\infty}(B\stackrel{id}{\longrightarrow}B)=L_{B}\}$, here $\Sigma^{\infty}$ is the infinity suspension functor from $CAlg_{/B}$ to $Sp(CAlg_{/B})=Mod(B)$. So actually this corresponds to the infinity suspension functor acting on the pushout diagram of the (unreduced) suspension on $A\rightarrow B$, which is again a pushout diagram:
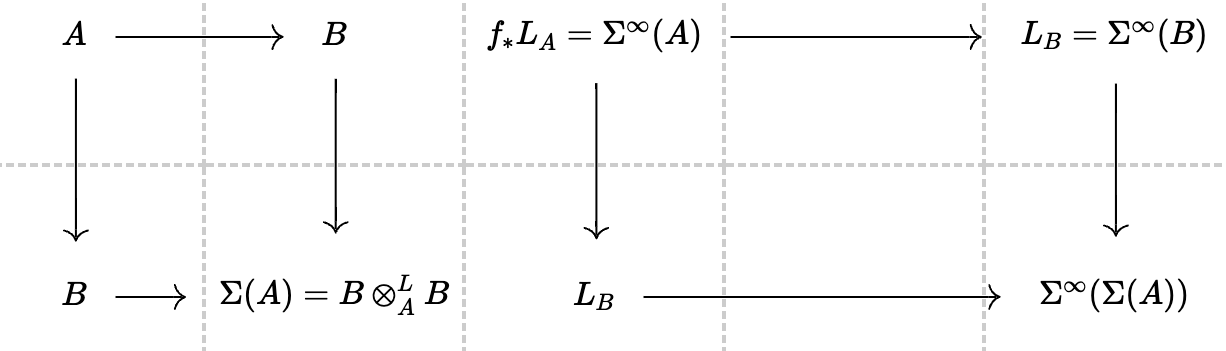(This observation probably has nothing to do with this question.)
$\textbf{Remark 2:}$ I do know that there is a $\textbf{Postnikov tower}$ of 'square zero extension', but I just don't know how it becomes a $\textbf{Goodwillie Tower}$ now.
Anyway, I guess this 1 excisive approximation should transfer between $CAlg_{/B}$ and its 'tangent space' $Sp(CAlg_{/B})=Mod(B)$ and some 'linear' approximation happens in this tangent space, so it should be $\textbf{related}$ with $\textbf{infinite suspension}$ $\Sigma^{\infty}$ which sends things to the 'tangent space', $\textbf{infinite loop}$ which takes things back from tangent space, and the $\textbf{derivative}$ $\partial F$. So:
$\textbf{Question:}$ $\textbf{What is the relationship between $\varinjlim_{k}\Omega^{k}\circ \Sigma^{k}$ and $\Sigma^{\infty}$ along with $\Omega^{\infty}$?}$
There must be some way to pack them together, any references or ideas on this topic are all very welcome!
