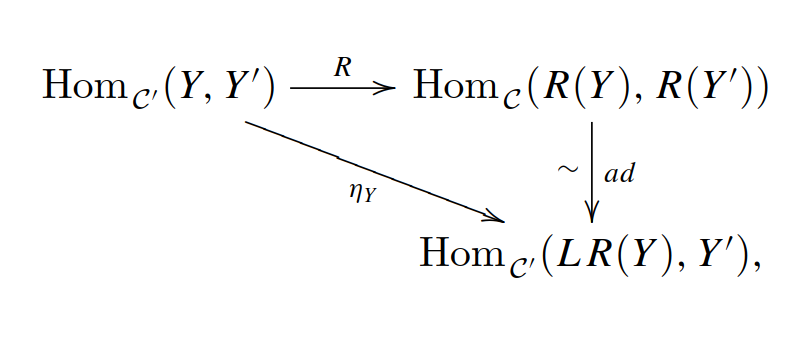$\DeclareMathOperator\Hom{Hom}\newcommand\id{\mathrm{id}}\newcommand\C{\mathcal C}$Right adjoint $R \colon \C' \to \C$ and left adjoint $L$.
$R$ is fully faithful iff the counit $\eta \colon LR \to \id_{\C'}$ is an isomorphism. The proof is given below from the textbook (Categories and Sheaves) using the commutative diagram.
Proof: The map $\Hom_{\C'}(Y,Y') \to \Hom_{\C}(RY,RY')$ is bijective iff the map $\Hom_{\C'}(Y,Y') \to \Hom_{\C}(LRY,Y')$. Therefore, $R$ is fully faithful iff the map $LRY \to Y$ is an isomorphism for all $Y$.
I know that this question has been answered in Right adjoint is fully faithful iff the counit is an isomorphism (without Yoneda).
I understand that, $R$ fully faithful, taking $Y'$ to be $LRY$ we get that $\eta_Y$ has a left inverse $g \colon Y \to Y'$. It was not clear how is $g$ also a right inverse. I somewhat know the explanation has to be simple but I am being foolish about it.