I am going through this paper by Tanaka, but I got stuck at Proposition 2.4, given below.
He does not provide any proof, instead referring to Theorem 6.6 of this paper, given below. 
Unfortunately, I cannot figure out how the Proposition follows from Theorem.
Can anyone provide any hints or suggestions? They will be really appreciated.
Thanks and regards in advance.
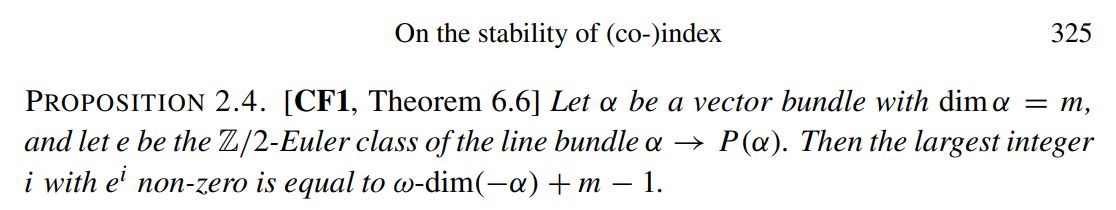
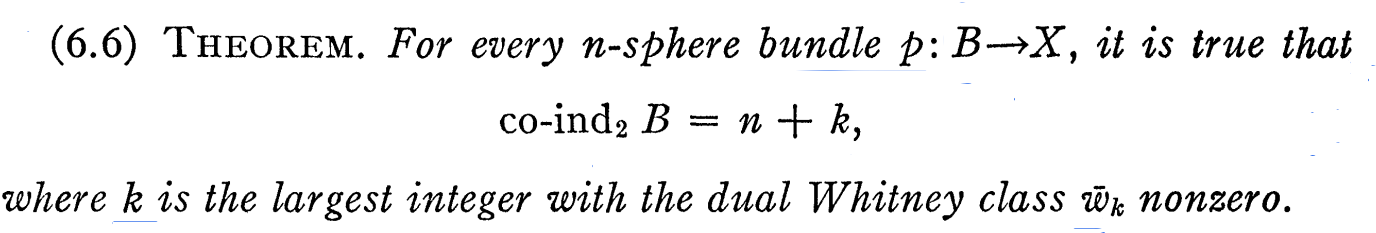Given a finite group $G$ acting freely on a paracompact space $X$, a principal ideal domain $L$, and a positive integer $n$, Conner and Floyd construct a cohomology class $c^n(X; L)$ on $X/G$ (see Section 4). If $G = L = \mathbb{Z}_2$, then $c^n(X; L) = w_1(\ell)^n$ where $\ell$ is the real line bundle associated to the double cover $X \to X/\mathbb{Z}_2$. They then define $\operatorname{co-ind}_LX$ to be the largest integer $n$ such that $c^n(X; L) \neq 0$ (see Definition 4.4); furthermore, $\operatorname{co-ind}_{\mathbb{Z}_2}X$ is denoted by $\operatorname{co-ind}_2X$. In particular, if $G = L = \mathbb{Z}_2$, then $\operatorname{co-ind}_2X$ is the largest integer $n$ such that $w_1(\ell)^n \neq 0$.
In Section 6, Conner and Floyd consider the total space of an $n$-sphere bundle $p : B \to X$ with structure group $O(n+1)$ - note, the $n$ used here is unrelated to the $n$ used in the previous paragraph. Since the structure group is $O(n+1)$, there is a rank $n+1$ vector bundle $\alpha \to X$ with $B = S(\alpha)$. Note that the antipodal map on each fiber of $p$ is a free involution on $B$, and $B/\mathbb{Z}_2 = S(\alpha)/\mathbb{Z}_2 = \mathbb{P}(\alpha)$. Theorem 6.6 states that $\operatorname{co-ind}_2B = n + k$ where $k$ is the largest integer such that the dual Stiefel-Whitney class $\overline{w}_k(\alpha)$ is non-zero. So, if $\ell \to \mathbb{P}(\alpha)$ denotes the real line bundle corresponding to $S(\alpha) \to \mathbb{P}(\alpha)$, then the largest $i$ for which $w_1(\ell)^i \neq 0$ is $i = n + k$.
Converting these results to Tanaka's statement, note that Tanaka is instead considering a vector bundle $\alpha$ of rank $m$ (so $n = m - 1$), and is using $e$ to denote $w_1(\ell)$. Tanaka defines $\omega\operatorname{-dim}(\alpha)$ to be the largest integer $k$ for which $w_k(\alpha)$ is non-zero. Note that $-\alpha$ is a virtual vector bundle with $\alpha\oplus(-\alpha)$ trivial, so $w(\alpha)w(-\alpha) = 1$, and hence $w(-\alpha) = \overline{w}(\alpha)$. Therefore $\omega\operatorname{-dim}(-\alpha)$ is the largest integer $k$ for which $w_k(-\alpha) = \overline{w}_k(\alpha)$ is non-zero - Conner and Floyd simply called this value $k$, i.e. $\omega\operatorname{-dim}(-\alpha) = k$. Putting this all together, we see that the largest $i$ for which $e^i = w_1(\ell)^i$ is non-zero is
$$\operatorname{co-ind}_2(S(\alpha)) = n + k = m - 1 + \omega\operatorname{-dim}(-\alpha)$$
which yields Tanaka's Proposition 2.4.
