We are trying to prove the following statement for convex hyperbolic pentagons which we believe should be true.
Consider a convex hyperbolic pentagon with sides of lengths $a, b, c, d, e$. Suppose the interior angles labelled as $\alpha, \beta, \gamma, \delta, \nu$. If ($\alpha + \beta + \delta$) and ($\gamma+\nu$) are given, then the pentagon is determined uniquely.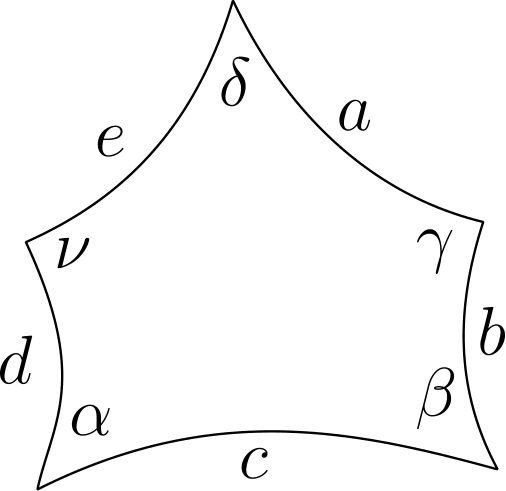
In trying to prove this, we used the following lemma:
Lemma: Suppose the convex hyperbolic pentagon as in the picture above. If all the side lengths are given and any two angles are known then the pentagon is uniquely determined.
First we treat $\gamma$ as a variable. The second angle sum condition determines $\nu$ as a function of $\gamma$. By the above lemma and by using only the second angle condition, we get a one parameter family of pentagons. Now, the goal is to show that the first angle sum condition determines the pentagon uniquely. We tried to show this by proving that the first angle sum is an increasing/decreasing function of $\gamma$ but the argument involved very cumbersome hyperbolic trigonometric functions which we could not handle. Is there a nice way to prove or disprove this statement?
