A question about rigid objects in monoidal categories

Question

Let $(\mathcal{A},\otimes,1_{\mathcal{A}})$ be a monoidal category, and let $M$ be a rigid object of $M$, with left and right dual respectively denoted by $$ \Big\{M^*,~~~ \epsilon_l:M^* \otimes M \to 1_{\mathcal{A}},~~~ \eta_l:1_{\mathcal{A}} \to M \otimes M^*\Big\} $$ and $$ \Big\{{}^*M, ~~~\epsilon_r: M \otimes {}^*M \to 1_{\mathcal{A}},~~~\eta_r: 1_{\mathcal{A}} \to {}^*M \otimes M\Big\} $$ respectively (just as in Wikipedia $\epsilon$ denotes evaluation and $\eta$ the coevaluation). Let's assume that we have isomorphisms $f_l:M \to M^*$ and $f_r:M \to {}^*M$. Then do we have the identity $$ (\mathrm{id} \otimes f_l^{-1}) \circ \eta_l(1_{\mathcal{A}}) = (f_r^{-1} \otimes \mathrm{id}) \circ \eta_r(1_{\mathcal{A}})? $$ This works for finite dimensional vector spaces, so I have guessed that it works in general. Also, do we have the identities $$ \epsilon_l \circ (f_l \otimes \mathrm{id}) = \epsilon_r \circ (\mathrm{id} \otimes f_r): M \otimes M \to 1_{\mathcal{A}}? $$

Answer 1

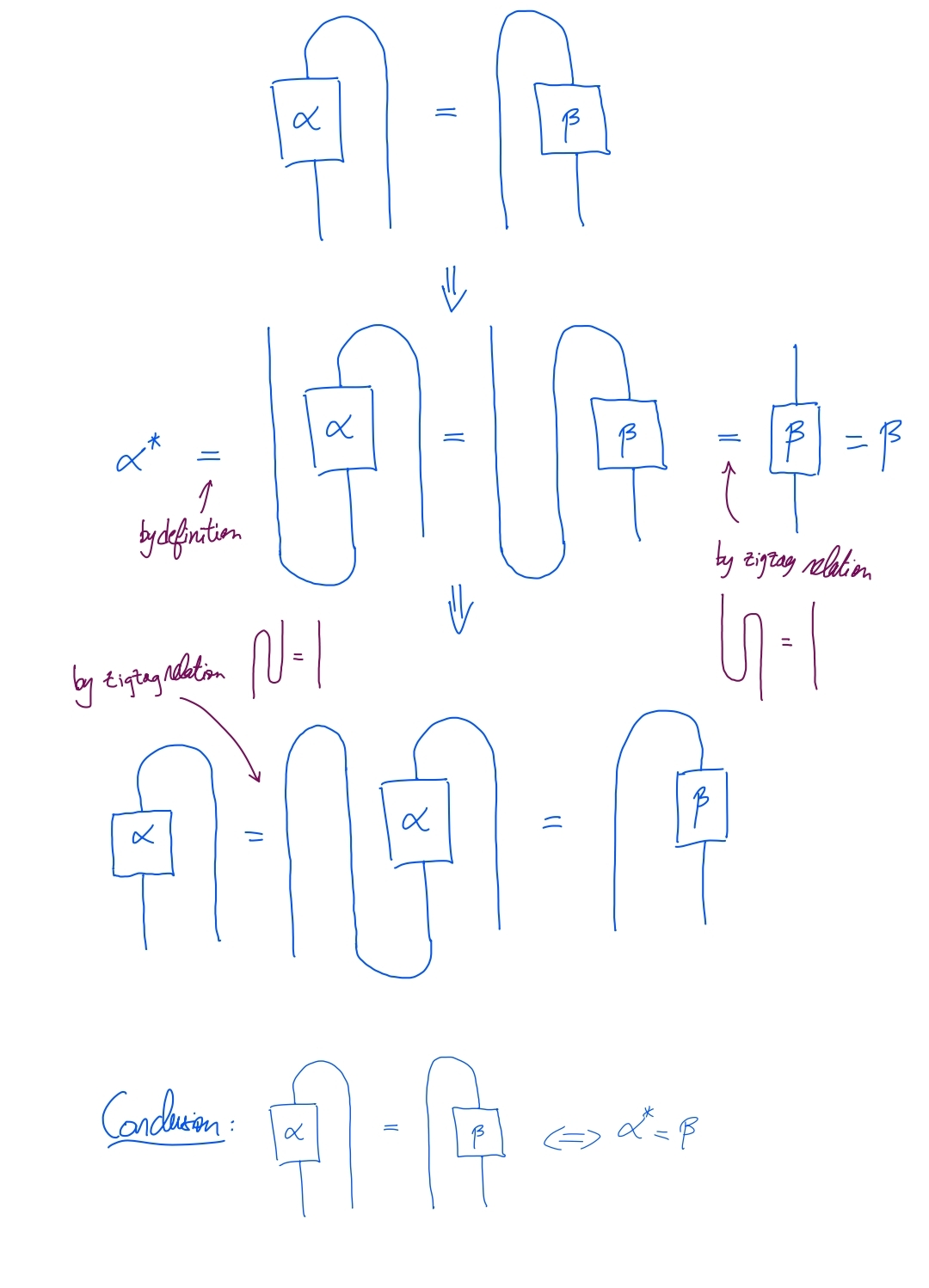
Below is a graphical calculus:

For more details, you can read Sections 2 and 7 of my preprint arxiv:2203.06522 or watch my course of current semester on YouTube, from Session 3 at 15:00 here, and from Session 10 at 43:00 there.