19
$\begingroup$

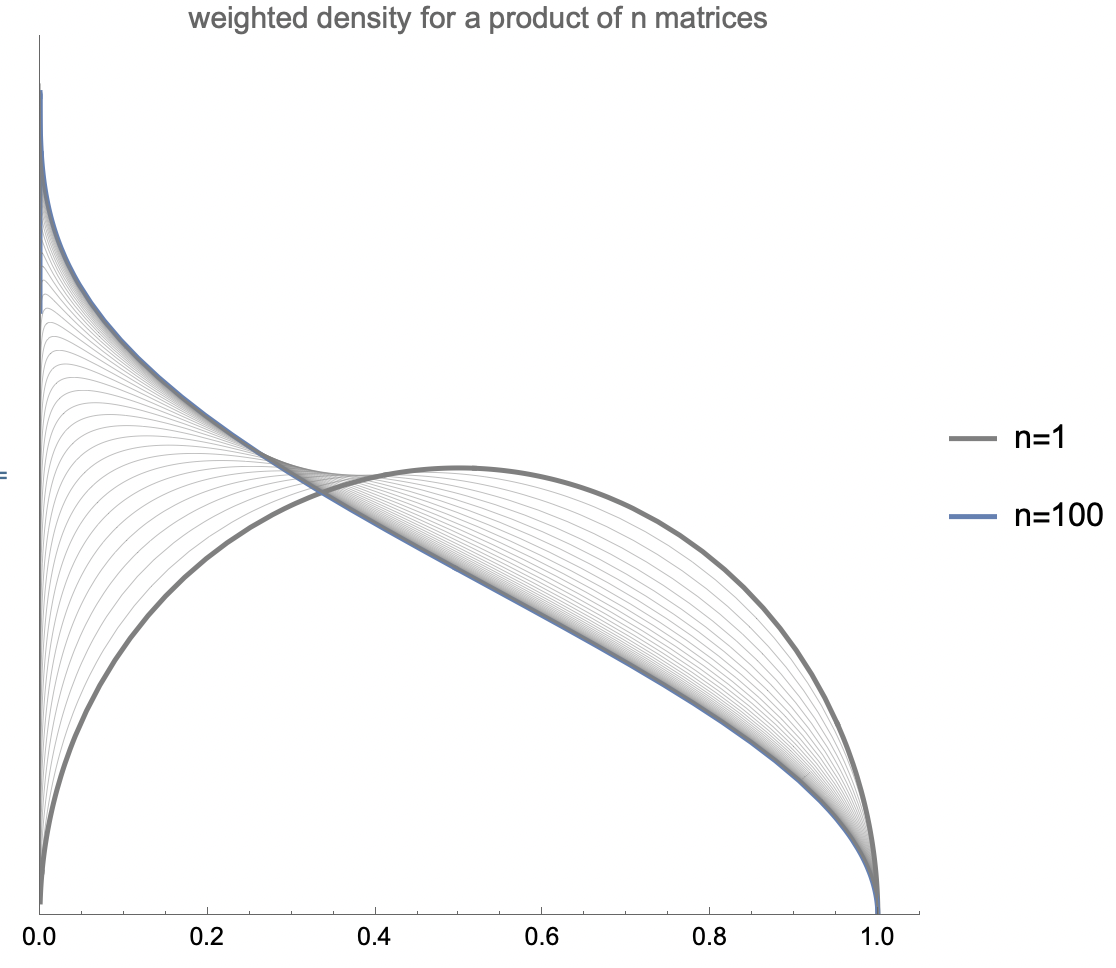
Let $A$ be a product of $n$ $d\times d$ matrices with IID standard Gaussian entries and consider the value of $g(x)=x f(x)$ where $f(x)$ is the density of squared singular values of $A/\|A\|$.

Is anything known about $g(x)$ in the $n\to \infty$, $d\to \infty$ limit, where $d$ limit taken first?

The effect of taking $n\to \infty$ limit first is better understood as such product is almost surely rank-1. Plotting $g(x)$ heuristically by relying on formula 3.61 from Ipsen's thesis yields the chart below:

enter image description here

Notebook

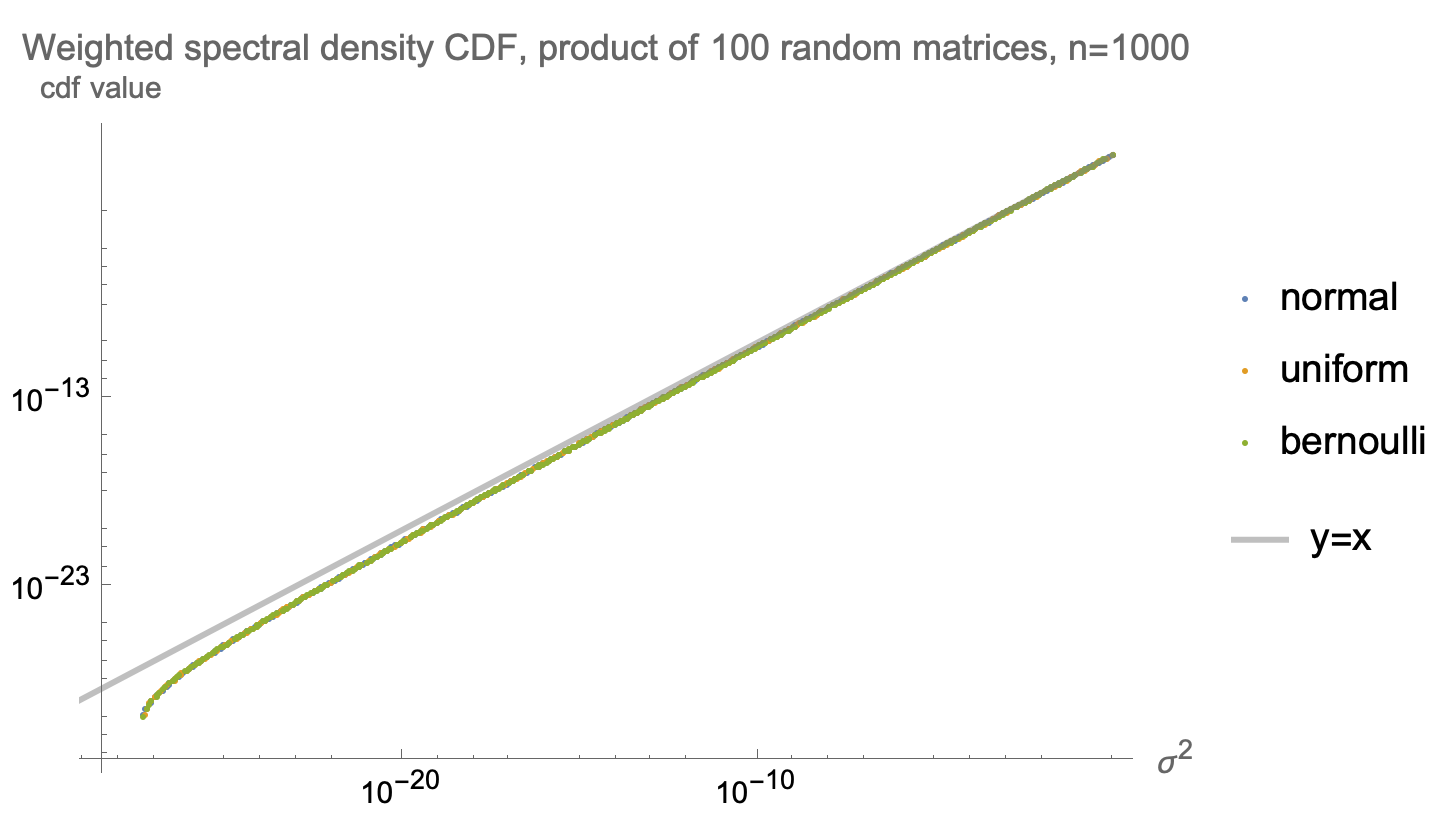
Empirically, I see the same density when considering matrices with Gaussian, symmetric Bernoulli and symmetric uniform entries so I'm wondering if the large $n$ limit has a name.

This

Improve this question
$\endgroup$
6
  • 2
    $\begingroup$ This is a slightly different problem (matrices are rectangular and main results is when outer dimension is small) but may be helpful cs.cmu.edu/afs/cs/user/dwoodruf/www/lw21.pdf $\endgroup$
    – EvgeniyZh
    Commented Aug 8, 2023 at 8:49
  • $\begingroup$ Motivated by your added "free-probability" tag: To use multiplicative free convolution via the S-transform, the mean of the eigenvalue distribution would have to be positive - one could imagine shifting the semicircular distribution by a small amount $\epsilon $. Then, the S-transform can be given and taken to the $n$-th power. Re-extracting the corresponding eigenvalue distribution doesn't seem straightforward - I haven't made further progress in that direction. If you're interested in such a partial/failed attempt, I'll be happy to post it ... maybe someone sees how to take it further. $\endgroup$
    – Michael Engelhardt
    Commented Aug 9, 2023 at 2:22
  • $\begingroup$ @MichaelEngelhardt S-transform of $f(x)$ is $\frac{1}{(1+z)^n}$, right? (Eq 32 of Burda). What's not clear to me is 1) why does $\frac{1}{(1+z)^\infty}$, give $1/x$ behavior at 0 and 2) how to get S-transform of $g(x)$ $\endgroup$
    – Yaroslav Bulatov
    Commented Aug 9, 2023 at 2:55
  • 1
    $\begingroup$ This formula of Burda is for the product of Wishart matrices, i.e., squares of Gaussian matrices. That seems to me to be different from what you're considering. For a Gaussian matrix, the S-transform is $1/\sqrt{z} $, and you'll have trouble inverting something like $1/z^{n/2} $. Hence the need for a shift by $\epsilon $ ... $\endgroup$
    – Michael Engelhardt
    Commented Aug 9, 2023 at 3:23
  • $\begingroup$ @MichaelEngelhardt I've checked formula 35 which is density that Burda gets by inverting $\frac{1}{(1+z)^2}$ S-transform, and it matches Ipsen's formula 3.61 for eigenvalues of $XYY'X'$, which is squared singular values of $XY$, Gaussian $X,Y$ $\endgroup$
    – Yaroslav Bulatov
    Commented Aug 9, 2023 at 6:05

0

Reset to default

You must log in to answer this question.