0
$\begingroup$

If we have a function f that has a minimum at a point say x. Does f preserve the min if we convolve it with a mollifier?

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ I have voted to close this because this does not seem like a question that could arise in research. It looks more like a homework problem, and a rather easy one at that. Sorry to be so blunt about it – please check out the first two questions in the faq to see what mathoverflow is all about. $\endgroup$
    – Harald Hanche-Olsen
    Commented Nov 6, 2010 at 22:00
  • $\begingroup$ Oh! It wasn't a home work problem. I have been reading about convolutions and thought of this question... In any case thank you for your time! $\endgroup$
    – PDE
    Commented Nov 6, 2010 at 22:18
  • 2
    $\begingroup$ Even it is not homework, it is a good question to try to answer on your own. $\endgroup$
    – Deane Yang
    Commented Nov 6, 2010 at 23:07
  • 1
    $\begingroup$ I agree with Deane Yang; it's important to exercise your brain before asking for help. It would be worth the effort to consider different mollifiers or smoothing functions and attempt to calculate and understand what the convolution of the mollifier with $f$ would result in. I didn't even know the word mollifier as a mathematical term until just 2 minutes ago; however, vocabulary expansion alone is not a good reason for a question to be on mathoverflow. I'd vote to close this question also, if I had the points to do it. $\endgroup$
    – sleepless in beantown
    Commented Nov 7, 2010 at 3:11
  • $\begingroup$ This question is begging for a more specific title. $\endgroup$
    – j.c.
    Commented Nov 7, 2010 at 12:53

1 Answer 1

Reset to default
1
$\begingroup$

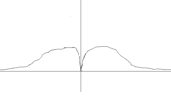
alt text
(source: uci.edu)

No, consider a function $f$ with a graph something like the above for example. (Pardon the rough sketch:-) When you mollify it the minimum at the origin will rise and become greater that the value of the mollified function away from the origin.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I see that you answered a different question than I thought was being asked. Now that I think of it, I can come up with at least three different interpretations of the question. Not that this changes my opinion that the question should be closed, however. $\endgroup$
    – Harald Hanche-Olsen
    Commented Nov 6, 2010 at 22:17

Not the answer you're looking for? Browse other questions tagged .