A claim on concurrency of 'Width Bisectors' of planar convex regions

Question

We add a bit to A claim on the concurrency of area bisectors of planar convex regions

Define a width of a planar convex region $C$ as the distance between two parallel lines that just touch $C$. A width bisector is a line that is parallel to a pair of parallel lines that are tangent to $C$ and is at same distance from both these lines - basically, it divides a width into two equal parts.

Question: is this claim valid: "The width bisectors of a planar convex region $C$ are concurrent if and only if $C$ is centrally symmetric."

Only the converse needs to be proved.

Some more on this is at https://nandacumar.blogspot.com/2023/06/lines-segmenting-convex-planar-regions.html

Answer 1

We should assume that $C$ is closed; otherwise, there are easy counterexamples. If $C$ is unbounded, then there is at most one width bisector, and hence easy counterexamples again.

So, assume that $C$ is a compact convex set with all width bisectors passing through a point $O$.

Without loss of generality, the interior of $C$ is nonempty (otherwise, the desired conclusion is obvious).

Note that $O$ is in the interior of $C$ -- otherwise, there will be a (straight) line $l$ non-strictly separating $O$ from $C$, and hence the width bisector of $C$ parallel to $l$ would not pass through $O$.

Now, suppose first that $C$ is smooth, in the sense that through each boundary point of $C$ there is only one line supporting $C$. Let $AB$ be a chord in $C$ through $O$ with the maximal value, say $r_{\max}$, of the ratio $|BO|/|AO|$. Suppose that $r_{\max}>1$. Then the lines, say $l_A$ and $l_B$, through the boundary points $A$ and $B$ supporting $C$ cannot be parallel (otherwise, the width bisector parallel to $l_A$ and $l_B$ would not pass through the point $O$). So, it is easy to show (see details below) that one can rotate the chord $AB$ slightly clockwise or counterclockwise about $O$ to get a new chord $A_1B_1$ of $C$ through $O$ with the ratio $|B_1O|/|A_1O|>|BO|/|AO|=r_{\max}$, a contradiction. So, $r_{\max}$ is $\le1$ and hence $=1$. That is, $C$ is centrally symmetric.

If now $C$ is not smooth, smooth it up by considering $C_t:=C+tD$ for real $t>0$, where $D$ is the unit disk. Note that $C_t$ is smooth and has the same bisectors as $C$ -- which follows because, for any unit vector $u$ we have $\max(u\cdot C_t)=\max(u\cdot C)+\max(u\cdot(tD))=\max(u\cdot C)+t$, where $\cdot$ denotes the dot product and $u\cdot E:=\{u\cdot x\colon\, x\in E\}$. So, by what was shown, $C_t$ is centrally symmetric, for each real $t>0$. So, $C=\bigcap_{t>0}C_t$ is centrally symmetric. $\quad\Box$

---

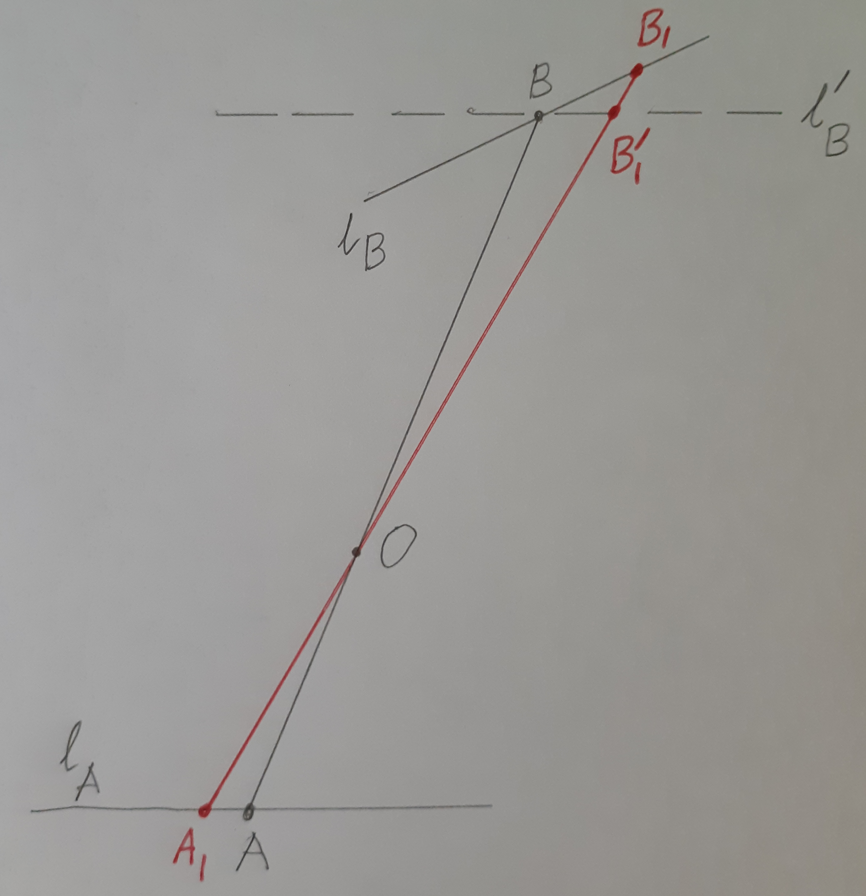
Details on the inequality $|B_1O|/|A_1O|>|BO|/|AO|$: In the picture below, as in the setting above, $AB$ is a chord in $C$ through $O$, and $l_A$ and $l_B$ are the lines through the boundary points $A$ and $B$ supporting the smooth convex set $C$, and $l_A$ and $l_B$ are not parallel. The dashed line $l'_B$ is the line through $B$ parallel to $l_A$. The segment $A_1B_1$ is a chord of $C$ through $O$ close to the chord $AB$ of $C$, so that $A_1$ is almost on the line $l_A$ and $B_1$ is almost on the line $l_B$, since $C$ is smooth. Also, $B_1$ is "above" the line $l'_B$. The point $B'_1$ is the point of intersection of the line $l'_B$ with the line through $A_1$ and $B_1$. It is clear now that $|B_1O|/|A_1O|>|B'_1O|/|A_1O|=|BO|/|AO|$.

---

Almost the same reasoning proves the natural generalization of this "planar" result to the corresponding one for any dimension.

Answer 2

$\newcommand\de\delta\newcommand\R{\mathbb R}$As in the previous answer, assume that $C$ is a compact convex subset of $\R^2$. The condition that all the width bisectors of $C$ are concurrent means that the support function $\de^*_C$ of $C$ is even, where $$\de^*_C(x^*):=\sup\{x\cdot x^*\colon x\in C\}$$ for $x^*\in\R^2$ and $\cdot$ is the dot product.

By (say) Theorem 13.1, $$x\in C\iff \forall x^*\in\R^2\ x\cdot x^*\le\de^*_C(x^*).$$ So, for any $x\in C$ and any $x^*\in\R^2$ we have $$(-x)\cdot x^*=x\cdot(-x^*)\le\de^*_C(-x^*)=\de^*_C(x^*),$$ so that $-x\in C$. Thus, $C$ is centrally symmetric. $\quad\Box$

---

Again, almost the same reasoning proves the natural generalization of this "planar" result to the corresponding one for any dimension.