Geometric proof of the three-dimensional Pythagorean theorem

Question

All the proofs of the high-dimensional Pythagorean theorem that I know are based on induction or the additivity of the dot product. Is there any geometric construction that's similar to the well-known planar proofs, ones that are based on clever divisions of squares or similarities between certain triangles?

Answer 1

In a rectangular parallelepiped with edges $|AB|=a$, $|BC|=b$, $|CD|=c$ and space diagonal $|AD|=d$ let us draw altitudes $BX$ and $CY$ from the vertices to $AD$. Then using similarity arguments it is easy to see that $|AX|=a^2/d$ and $|YD|=c^2/d$. Using, say, a parallel translation and a similarity argument one also sees that $|XY|=b^2/d$. We obtain $a^2/d+b^2/d+c^2/d=d$. This is probably in some sense dual to what you wrote in your comment.

Answer 2

From the comments, it appears that the generalization in the $n$-dimensional space should consider codimension one faces. The answer is given from this point of view.

---

The suggested version in the $n$-dimensional space:

Consider $O,A,B,\dots ,Z$ to be $(n+1)$ points in the euclidean $n$-dimensional space, so that each two of the segments $OA$, $OB$, ... , $OZ$ are forming a right angle. Consider $\Pi$ to be the $n$-dimensional solid with these vertices. (Convex hull.) Its faces are $\Pi_O$, $\Pi_A$, $\Pi_B$, ... , $\Pi_Z$. And let $h_O$; $h_A,h_B,\dots,h_Z$ be the corresponding heights build each from the remained vertex not in the face. For this, let $H$ be the projection of $O$ on $\Pi_O$. (All other projections are $O$.)

We can then build $(n-1)$-dimensional volumes of the faces, and $1$-dimensional volumes (lengths) of the heights. Denote volumes (in appropriate dimension) by $v$ Then, given the formula for the $\Pi$-volume in terms of using the one or the other face, we have two equivalent formulations: $$ \begin{aligned} v(\Pi_O)^2 &= v(\Pi_A)^2+v(\Pi_B)^2+\dots + v(\Pi_Z)^2\ ,\\ \frac 1{h_O^2} &=\frac 1{h_A^2} + \frac 1{h_B^2} + \dots + \frac 1{h_Z^2}\ . \end{aligned} $$

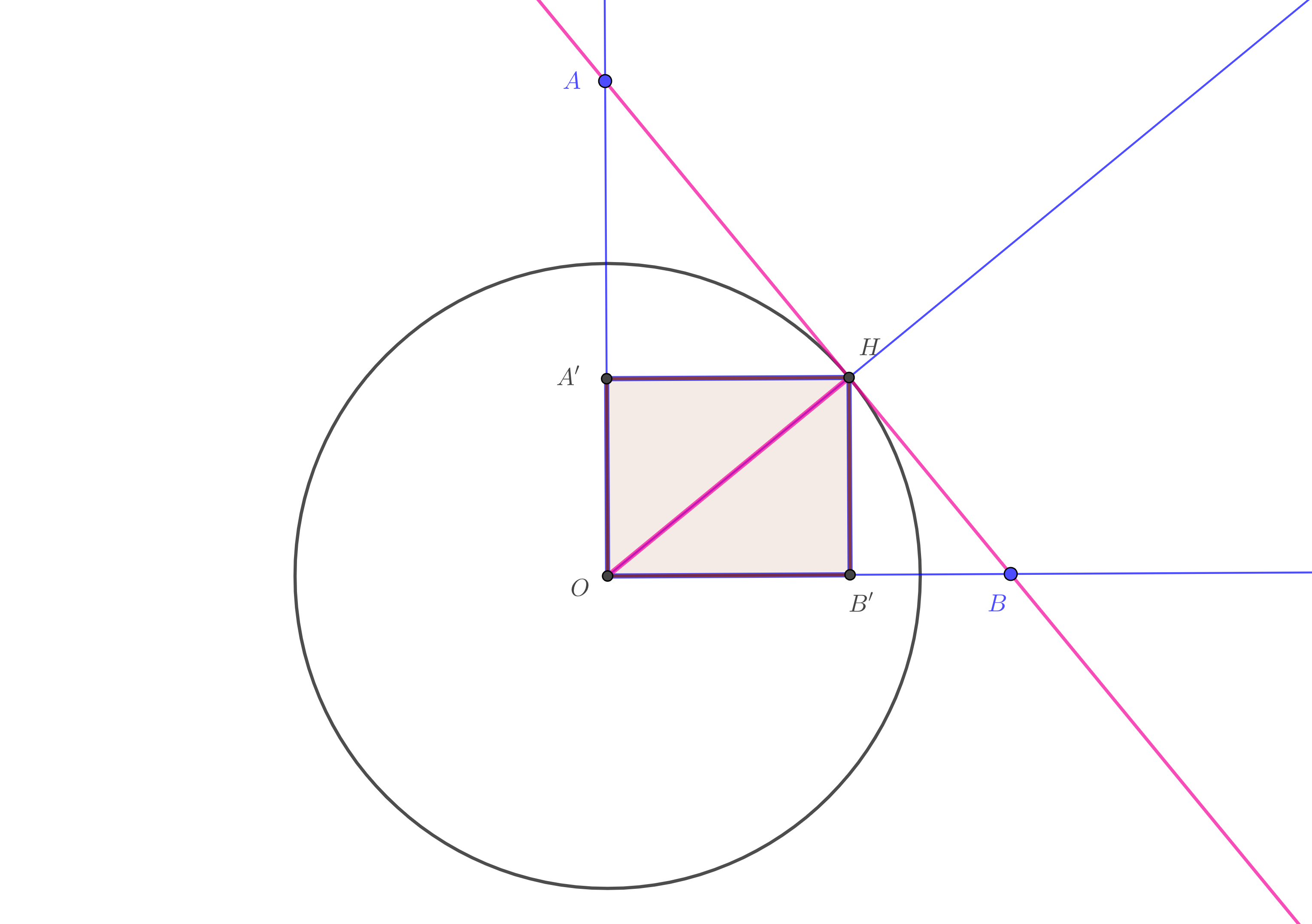
Let us show the second version by inversion. Which arguably involves similarities, from right triangles like $\Delta OAH$ we obtain, building the inverses $A',H'$ also right triangles like $\Delta OA'H'$. The inversion, denoted by a prime, is centered in $O$. The plane of the face $\Pi_O$ is mapped into a sphere. The two dimensional picture is (taking the power of the inversion to be $OH^2$, so that $H'=H$):

The $n$-dimensional picture is similar, the "box" determined by $O;A',B',\dots,Z';H=H'$ is an $n$-dimensional cartesian product of intervals.

The relation to be shown for the heights becomes explicity, step by step: $$ \begin{aligned} \frac 1{h_O^2} &=\frac 1{h_A^2} + \frac 1{h_B^2} + \dots + \frac 1{h_Z^2}\ , \\ \frac 1{OH^2} &=\frac 1{OA^2} + \frac 1{OB^2} + \dots + \frac 1{OZ^2}\ , \\ {OH'}^2 &={OA'}^2 + {OB'}^2 + \dots + {OZ'}^2\ , \end{aligned} $$ so the $(n-1)$-dimensional Pythagoras is dual to the $1$-dimensional Pythagoras.

$\square$

---

Note: In the picture, relations like $OA\cdot OA'=OH^2$ are also used to show the theorem in elementary books.