By a Tarski space I understand a mathematical structure $(X,\mathsf B,\equiv)$ consisting of set $X$, a betweenness relation $\mathsf B\subseteq X^3$ and a congruence relation ${\equiv}\subseteq X^2\times X^2$ satisfying all Tarski's axioms except for the Euclid Axiom, the Axiom of Continuity, and the Dimension Axioms. In other words, Tarski spaces should satisfy seven Tarski's axioms: (1) Reflexivity of Congruence, (2) Identity of Congruence, (3) Transitivity of Congruence, (4) Identity of Betweenness, (5) Five Segments, (6) Segment Construction, (7) Inner Pasch Axiom. A Tarski space is called a Tarski plane if it has affine dimension 2.
As shown in the book of Schwabhäuser, Szmielew, and Tarski, a substantial portion of familiar (absolute) geometry holds in Tarski spaces of dimension $\ge 2$. In particular, one can construct midpoints and right triangles with given sides. By definition, a point $m$ is the midpoint between points $x$ and $y$ if $\mathsf Bxmy$ and $xm\equiv my$. Given three points $x,o,y$ of a Tarski space $X$, we write $\mathsf Rxoy$ and say that the angle $\angle xoy$ is right if $xy\equiv \dot xy$ where $\dot x$ is a unique point such that $o$ is the midpoint between $x$ and $\dot x$.
The geometric constructions in a Tarski space correspond to constructions with the help of a straightedge and a divider (the latter tool can be used to measure and transfer distances on lines, but not to draw circles).
Problem 1. Is an equilateral tringle constructible in a Tarski plane?
Remark. An equilateral triangle can be easily constructed in a Tarski space of dimension $\ge 3$: since we can construct perpendiculars to lines and planes in a Tarski space of dimension $\ge 3$, we can choose points $o,a,b,c$ such that $oa\equiv ob\equiv oc$ and $\mathsf Raob$, $\mathsf Raoc$ and $\mathsf Rboc$. Then the triangle $abc$ is equilateral.
In fact, we can mimic this construction also in dimension 2:
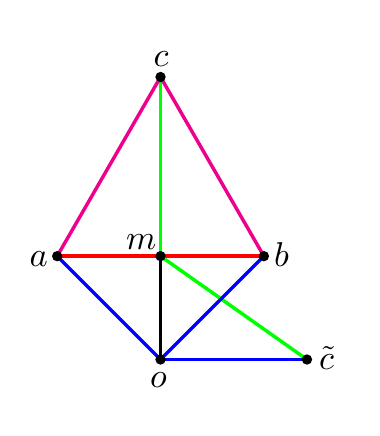
First, using a straightedge and a divider, construct points $a,o,b$ such that $ao\equiv ob$ and $\mathsf Raob$; then find the midpoint $m$ between the points $a$ and $b$. Construct a right triangle $mo\tilde c$ with sides $mo$ and $o\tilde c\equiv oa$. Finally take any point $c$ such that $\mathsf Rcma$ and $cm\equiv o\tilde c$.
Problem 2. Is the triangle $abc$ equilateral? Equivalently, can the congruence $ac\equiv ab$ be proved using only the seven axioms of a Tarski space?
This indeed can be done if the dimension of the Tarski space is $\ge 3$. This observation shows that Problems 1 and 2 are related to this problem.