Trace formula for monodromy of Milnor fibrations

Question

I am reading the paper A. Campo, Le nombre de Lefschetz d'une monodromie but I am stuck at several points, hope that someone can help me.

Let $P:\mathbb{C}^{n+1} \longrightarrow \mathbb{C}$ be a germ of holomorphic maps such that $P(0)=0$. Let $H = \left \{P = 0 \right \}$ be a hyperplane defined by $P$. Let $S_{\epsilon}$ be the $(2n+1)$-dimensional sphere of radius $\epsilon$. Then one knows from a famous result of Milnor that the map $$p: S_{\epsilon} \setminus (H \cap S_{\epsilon}) \longrightarrow S^1, \ z \longmapsto \frac{P(z)}{\left|P(z) \right|}$$ is a topological fibration. For each $\theta \in S^1$, let $F_{\theta} = p^{-1}(\theta)$. Consider the map $\gamma: [0,1] \longrightarrow S^1, t \longmapsto e^{2\pi i t}$, for each $x \in p^{-1}(\gamma(0))$, then by the lifting theorem (which is known to be true for fibrations) there exists a unique lifting, called the monodromy $$f: F_{\theta} \longrightarrow F_{\theta}, \ x = \gamma_x(0) \longrightarrow \gamma_x(1)$$ and this is homeomorphisms; hence it induces an automorphism on cohomology ring $f^*:H^*(F_{\theta};\mathbb{C}) \overset{\sim}{\longrightarrow} H^*(F_{\theta};\mathbb{C})$. The main result asserts that if $dP(0) = 0$ then the Lefschetz number is zero, i.e., $$\wedge(f^*) = \sum_{p \geq 0}(-1)^{q}\mathrm{Trace}(f^q)=0.$$ Campo proved this theorem by making an use of resolution of singularities (others, like Loeser, used motivic integration) combining with Leray spectral sequence, here it comes to the point that I do not really understand: first he proved a particular case where $P = z_1^{a_1}\cdots z_k^{a_k}$ where $1 \leq k \leq n+1$ and $a_1 + \cdots + a_k \geq 2$. To handle, he deforms the fibration at $1$, namely, $F_1$ into a nice space $R$ such that $R$ is compact, $f(R) \subset R$ but the monodromy $f$ has no fixed point on $R$; and the results follows by Lefschetz's fixed point theorem. For the general case, the setting is quite long, but not that terrible

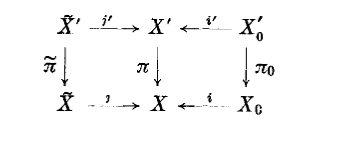
• $X$: analytic space, $x \in X$.
• $D$: disc, $D^{\circ} = D \setminus \left \{0 \right \}$: punctured disc.
• $P:(X,x) \longrightarrow (D,0)$ holomorphic.
• $F \in \mathbf{Sh}_c(X)$ a constructible sheaf of $\mathbb{C}$-vector spaces.
• $\widetilde{D^{\circ}} \longrightarrow D^{\circ}$ an universal covering space.
• $j: \widetilde{X} = X \times_D \widetilde{D^{\circ}} \longrightarrow X$ the projection.
• $i: X_0 = P^{-1}(0) \hookrightarrow X$.
• $\pi: X' \longrightarrow X$ a resolution of singularities ($\pi$ proper) and $\pi^{-1}(X_0) = X_0'$ is a normal crossing divisor in $X'$.
• $S = \pi^{-1}(0)$.

We have a commutative diagram

Set $F' = \pi^*F$, we consider the two nearby cycles functors $$\psi: D^b_c(X) \longrightarrow D_c^b(X_0), \ F \longmapsto i^* Rj_* j^*F$$ and $$\psi: D^b_c(X') \longrightarrow D_c^b(X_0'), \ F' \longrightarrow i'^*Rj_*' j^{'*}F'$$ (where by $D^b_c$ I mean the derived category of bounded complexes of constructible sheaves). They come with a monodromy action $T$. He claims that there is a spectral sequence $$E^{p,q}_2 = H^p(S, \psi^q(F')) \Longrightarrow E^{p,q}_{\infty} = \psi^{p+q}(F)_x,$$ and the monodromy $T$ acts on each page of this sequence. The original theorem is a special case of the following identity $$\sum_{q \geq 0} \mathrm{Trace}(T,(\psi^q(F))_x)=0$$ once we put $X = \mathbb{C}^{n+1}$ and $F = \underline{\mathbb{C}}$ the constant sheaf. So my questions are:

• Is there an easy to see why the monodromy action on nearby cycles coincides with the monodromy on Milnor fibrations?
• Why do we have this spectral sequence? I believe that this should be a version of Leray spectral sequence but I could not see how. And I think it should be $S = \pi^{-1}(x)$ because it makes no sense to write $\pi^{-1}(0)$.
• Next, he claims that $\wedge(T, (\psi(F'))_s)=0$ for all $s \in S$ and $F = \underline{\mathbb{C}}$ the constant sheaf. I do not see why do we need $F$ to be constant here as this should follow from the case $P=z_1^{a_1}\cdots z_k^{a_k}$.
• Finally, why do we deduce that $0 = \sum_{p,q \geq 0}(-1)^{p+q}\mathrm{Trace}(T,H^p(S,\psi^q(F')))$ just by knowing $\psi^*(F')$ are constructible sheaves?

Many thanks!