Let $f: \mathbb R^n \to \mathbb R$ be a locally integrable function.
Question: Suppose every point $x \in \mathbb R^n$ is a Lebesgue point of $f$. Does it follow that $f$ is continuous almost everywhere?
Note: We use the “strong” definition of Lebesgue point, given here.
As a warm-up, let's do an example with one point of discontinuity.
Our function $f$ looks like this:
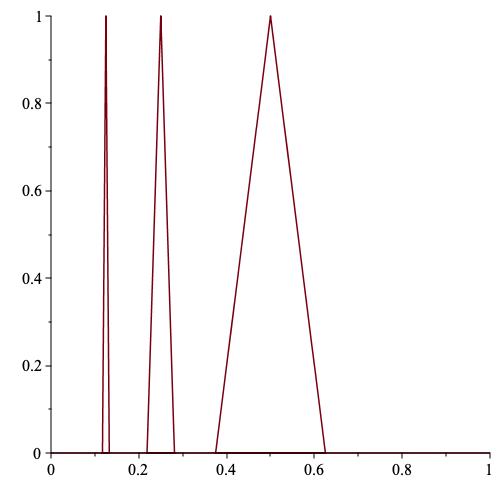
Here $f : \mathbb R \to \mathbb R$ is zero, except for a sequence of triangular spikes: height $1$, centered at $1/2^n, n=1,2,3,\dots$, with
width $1/4^n$, respectively.
Of course $f$ is continuous everywhere except $0$, and discontinuous at $0$. I claim every point is a Lebesgue point for $f$. By continuity, all nonzero points are Lebesgue points. What about $0$? For $r>0$,
$$ \frac{1}{\lambda(B_r(0))}\int_{B_r(0)}|f(0)-f(y)|\;dy =\frac{1}{2r}\int_0^r f(y)\;dy $$
Some simple estimates, $$ \int_0^1 f(y)\;dy = \frac12\sum_{n=1}^\infty\frac{1}{4^n} = \frac{1}{6} < \frac14 \\ \int_0^r f(y)\;dy \le \frac{1}{4}\qquad\text{for } \frac14 \le r \le\frac12 \\ \int_0^r f(y)\;dy \le \frac{1}{4^n}\qquad\text{for } \frac1{2^{n+1}} \le r \le\frac1{2^n}, n=1,2,3,\dots \\ \int_0^r f(y)\;dy \le 4r^2\qquad\text{for }0<r<\frac12 \\ \frac{1}{2r}\int_0^r f(y)\;dy \le 2r\qquad\text{for }0<r<\frac12 \\ \lim_{r\to 0^+}\frac{1}{2r}\int_0^r f(y)\;dy = 0 . $$
For the full question, I expect a counterexample will be almost the same. Let $E$ be a fat Cantor set. let $a_n$ be a sequence of points in the complement of $E$ that cluster everywhere in $E$; for example the centers of the open intervals removed to construct $E$. Then let $f$ be zero except for a sequence of triangluar spikes, height $1$, centered at $a_n$, width $b_n$, where $b_n \to 0$ very fast; in particular, $b_n \le \frac1{4^n}$ and $b_n < \frac14\operatorname{dist}(a_n,E)$. Proceed as above to show every point of $E$ is a Lebesgue point.
Here are some details on Sam Forster’s construction . To make the computation simpler I’d take powers of $4$ instead, i.e. define $g:= \sum_{k=1}^\infty \frac{f_k}{4^k},$ where $f_n$ and $C_n$ have been defined in his comment. For any $n$ we write $$g:= \sum_{k<n} \frac{f_k}{4^k}+ \frac{f_n}{4^n} +\sum_{k>n}^\infty \frac{f_k}{4^k}.$$ Taking into account Gerald Edgar’s construction we have the following. The first term is a linear combination of functions, all of them continuous in the open subset (of $[0,1]$), $[0,1]\setminus \bigcup_{k<n}C_k$. The function $f_n$ is discontinuous, with point-wise oscillation $\frac1{4^n} $, at any $x\in C_n$. The third term $\sum_{k>n}^\infty \frac{f_k}{4^k} $ has uniform norm bounded by $\sum_{k>n}^\infty \frac1{4^k} =\frac1{3\cdot4^n}$. So we have expressed $g$ as a sum of: a function which is continuous in a nbd of $S_n:=C_n \setminus \bigcup_{k<n}C_k$, a function with point-wise oscillation $\frac1{4^n}$ at any $x\in S_n\subset C_n$, and a function with uniform norm not larger than $\frac1{3\cdot4^n}$. Therefore for any $n$ the function $g$ has oscillation not less than $\frac1{4^n}-\frac2{3\cdot4^n}= \frac1{3\cdot4^n} $ at any $x\in S_n$, thus it is discontinuous on the full-measure set $\cup_nS_n$.
