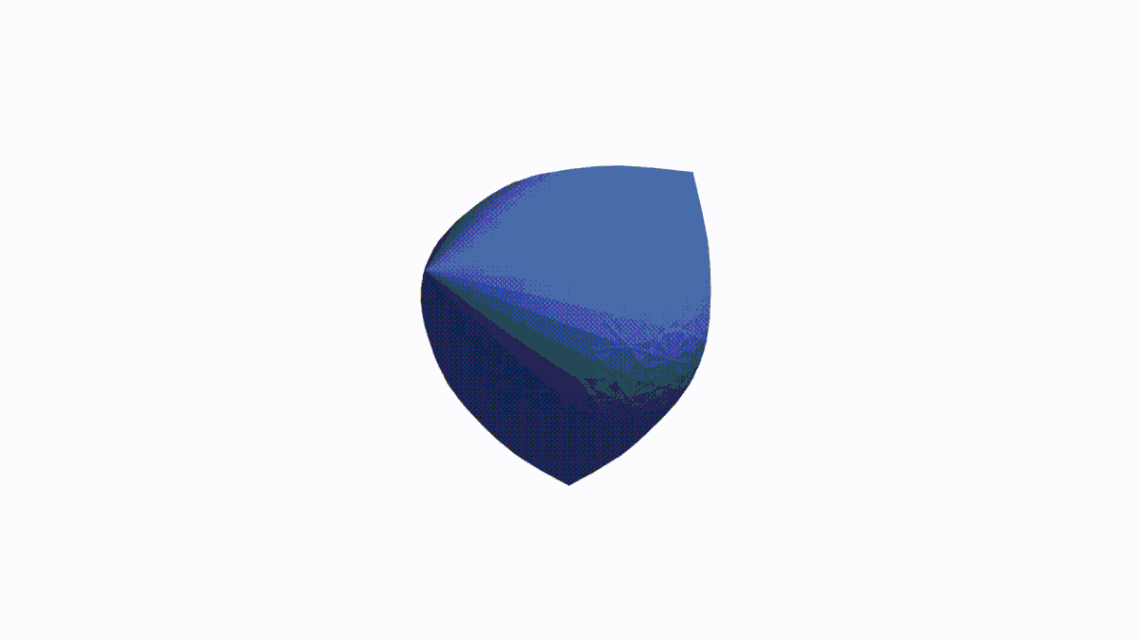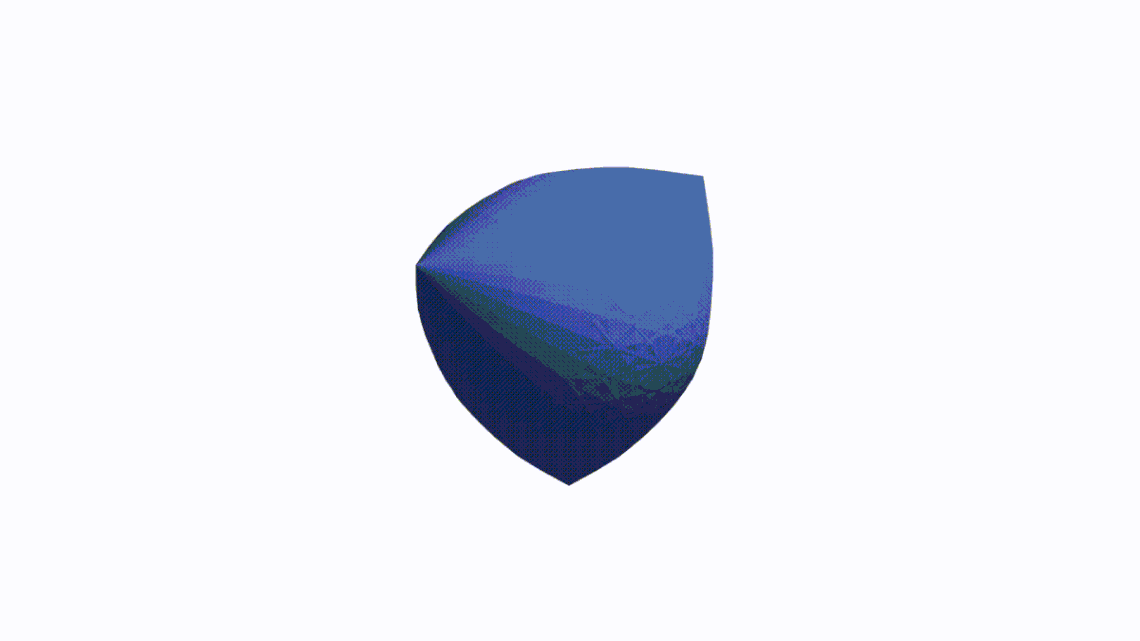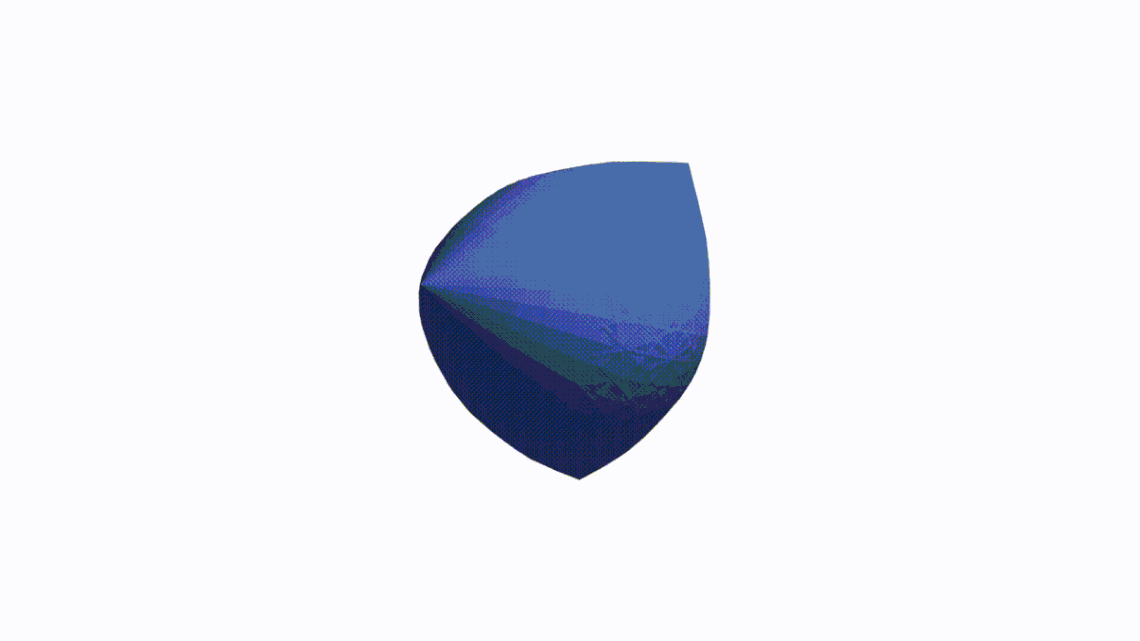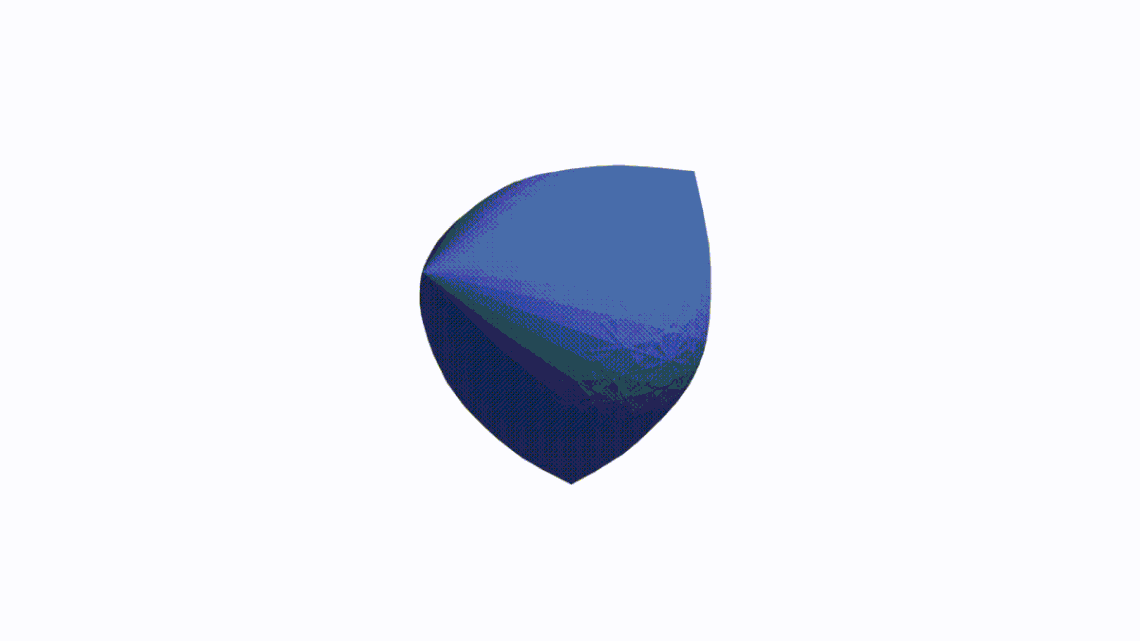
There are classical surfaces of revolution, shaped like footballs, that have constant positive curvature, except for their two cone points. How about such a surface with three cone points?
To give some context, a collaborator and I are trying to understand the moduli space of labeled quadrilaterals in $\mathbb{R}^3$ whose side lengths are all equal but whose joints are allowed to rotate freely. Kapovich and Millson showed that this moduli space is a Riemannian 2-manifold (in fact, a Kähler manifold), except for singular points corresponding to the three polygons that lie entirely along a single line. A fairly lengthy computation shows$^*$ that this manifold has constant positive curvature $1$; that each of the three singular points is an order-2 cone point; and that the distance from any cone point to any other is $\frac{\pi}{2}$. So the space looks something like the picture below, except that it has constant curvature.
It would be nice to have an isometric embedding of this space in $\mathbb{R}^3$. Since this is the simplest example of an elliptic orbifold with three cone points, we are hoping that there might be a known parametrization.
$^*$ For this, we scale the quadrilaterals to have unit perimeter, so their sides have length $\frac{1}{4}$.