A partial answer: a result of Juraj Bosák says that if all vertices of a graceful graph have even degree, then the graph has $4k$ or $4k+3$ edges for some integer $k$ (proof given below).
Since $K_5^{(3)}$ has $30$ edges, it is ungraceful.
Gallian's survey attributes this result to [Rosa 1967].
See also Don Knuth's work-in-progress section 7.2.2.3 of The Art of Computer Programming (https://www-cs-faculty.stanford.edu/~knuth/fasc7a.ps.gz) for a computational discussion graceful labelings as constraint satisfaction problems.
Lemma 7.2.2.3O from The Art of Computer Programming. In any graceful labeling of a graph with $4k+1$ or $4k+2$ edges, the number of vertices with an odd degree and an odd label is always odd.
Proof. We have $\sum_{uv\in E(G)}|l(u)-l(v)|=1+2+\dots+m=\binom{m+1}{2}$ when there are $m$ edges; and a given vertex $v$ appears exactly $\deg(v)$ times in this sum. Working modulo $2$, we also have $|l(u)-l(v)|\equiv l(u)+l(v)$. Therefore $\sum_v\deg(v)l(v)\equiv\binom{m+1}{2}$. But $\binom{m+1}{2}\equiv1$ when $m=4k+1$ or $m=4k+2$. $\square$
Overview: gracefulness of windmill graphs $K_n^{(j)}$
| $n$ $\backslash$ $j$ |
$1$ |
$2$ |
$3$ |
$4$ |
$5$ |
$6$ |
$7$ |
$j\ge8$ |
| $2$ |
✓ |
✓ |
✓ |
✓ |
✓ |
✓ |
✓ |
✓ |
| $3$ |
✓ |
✗ |
✗ |
✓ |
✓ |
✗ |
✗ |
✓ if and only if $j\equiv0\hbox{ or }1\pmod4$ |
| $4$ |
✓ |
✗ |
✗ |
✓ |
✓ |
✓ |
✓ |
✓ if $j\le1000$; ? otherwise |
| $5$ |
✗ |
✗ |
✗ |
✗ |
✗ |
? |
✗ |
✗ if $j$ is odd; ? if $j$ is even |
| $n\ge6$ |
✗ |
✗ |
✗ |
✗ |
✗ |
✗ |
✗ |
✗ |
When $n=2$, $K_2^{(j)}=K_{1,j}$ is a star and can always be gracefully labeled by placing $0$ in the internal vertex.
When $n=3$, $K_3^{(j)}$ is a friendship graph and is graceful if and only if $j\equiv0\hbox{ or }1\pmod4$.
The forward implication follows from J. Bosák's result in [Rosa 1965], and the reverse implication follows from a construction of [Skolem 1957].
When $n=4$ and $4\le j\le1000$, $K_n^{(j)}$ is graceful due to a construction of [Ge et al. 2010] (they construct $(12j+1,4,1)$-perfect distance families for $4\le j\le1000$, which are equivalent to graceful labelings of $K_4^{(j)}$).
When $j>1000$, nothing is known, though [Bermond 1979] conjectures that $K_4^{(j)}$ is graceful for $j\ge4$.
When $n=5$, if $j$ is odd, Bosák's result implies that $K_5^{(j)}$ is ungraceful. For even $j\ge6$, nothing is known.
When $n\ge6$, a result of [Koh et al. 1980] implies that $K_n^{(j)}$ is always ungraceful.
References
[Skolem 1957]
Thoralf A. Skolem,
On Certain Distributions of Integers In Pairs With Given Differences.
Mathematica Scandinavica 5 (1957), 57–68.
https://doi.org/10.7146/math.scand.a-10490
[Rosa 1965]
Alexander Rosa,
O Cyklických Rozkladoch Kompletného Grafu, Kandidátska dizertačná práca. (Bratislava: Českoslovanská akadémia vied, November 1965), ii+86 pages. (Note: Rosa attributes the result above to J. Bosák on page 17).
https://archive.org/details/o-cyklickych-rozkladoch-kompletneho-garfu
[Rosa 1967]
Alexander Rosa,
On certain valuations of the vertices of a graph.
Theory of Graphs (International Symposium, Rome, July 1966),
Gordon and Breach, N. Y. and Dunod Paris (1967), 349–355.
https://www.researchgate.net/publication/244474213_On_certain_valuations_of_the_vertices_of_a_graph
[Bermond 1979]
Jean-Claude Bermond,
Graceful graphs, radio antennae and French windmills.
In Graph Theory and Combinatorics (ed. R. J. Wilson),
Research Notes in Mathematics 34 (1979), 18–37.
(Proceedings of a one-day conference in combinatorics and graph theory held at the Open University, England, on 12 May 1978.)
https://hal.inria.fr/hal-02340680
[Koh et al. 1980]
Khee Meng Koh, D. G. Rogers, H. K. Teo, and K. Y. Yap,
Graceful graphs: some further results and problems.
Congressus Numerantium 29:
Proceedings of the 11th Southeastern Conference on Combinatorics, Graph Theory, and Computing, Winnipeg, Manitoba (December 1980), 559–571
[Ge et al. 2010]
Gennian Ge, Ying Miao, and Xianwei Sun,
Perfect difference families, perfect difference matrices and related combinatorial structures.
Journal of Combinatorial Designs 18(6) (2010), 415–449.
https://doi.org/10.1002/jcd.20259
[Knuth 2021]
Donald E. Knuth,
The Art of Computer Programming Volume 4 Pre-Fascicle 7A, Section 7.2.2.3: Constraint Satisfaction (2020–).
https://www-cs-faculty.stanford.edu/~knuth/fasc7a.ps.gz

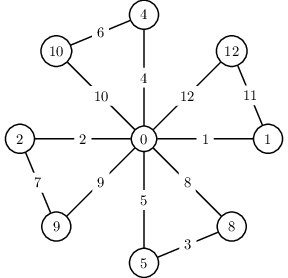 $K_3^{(4)}$." />
$K_3^{(4)}$." />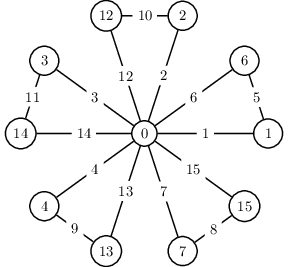 $K_3^{(5)}$." />
$K_3^{(5)}$." />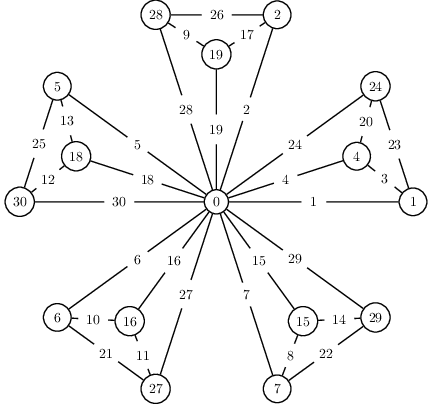 $K_4^{(5)}$." />
$K_4^{(5)}$." />