A block in a graph is a maximal connected subgraph that has no cut-vertex. A complete graph having $n$ nodes is denote by $K_n$. A block graph is a graph in which each block is a complete graph. For example in figure a block graph with blocks $K_2,K_2,K_3,K_4 $ and $K_5$. What will be determinant of adjacency matrix corresponding a general block graph?. 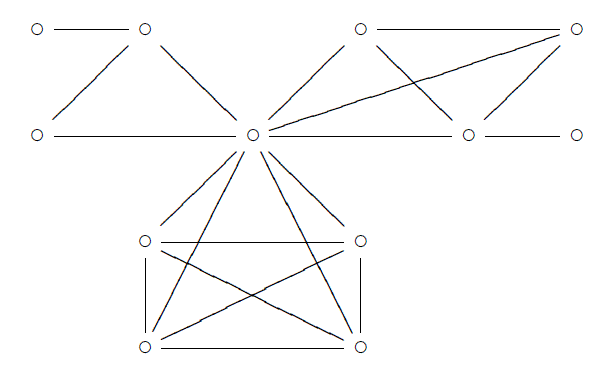
1 Answer
At first, it does not depend only the sizes of blocks (compare two trees on 4 vertices). At second, there is a recurrence for this value $\det(G) $coming from the leaf-block $B_0$ with $m$ vertices and a cut-vertex $v$: $$ \det(G)=(-1)^{m}(m-2)\det(G\setminus(B_0\setminus v))+(-1)^{m-1}(m-1)\det(G\setminus B_0). $$
Namely, if $B_0$ has vertices denoted by $1,\dots,m$, and vertex $m$ is a cut-vertex (so, this block $B_0$ corresponds to the northwest corner of the adjacency matrix), we may do the following.
0) if $m=2$, we get $\det(G)=-\det(G\setminus B_0)$ immediately.
1) Subtract first row from second, third,..., $m$-th rows.
2) Add second,third,..., $(m-1)$-th columns to the first column.
3) Subtract the first row divided by $(m-2)$ (assuming $m>2$) from the $m$-th row. Now the matrix has an upper-triangular block of size $(m-1)$ and the identity easily follows.
-
$\begingroup$ Can you please tell me from which row/column you are expanding the determinant. I have few comments (1) I think first term on RHS will have (-1)^(m-2) instead of (-1)^(m-1). (2) Matrix will have an upper triangular after your 2) step, to me step 3) looks redundant. $\endgroup$ Commented Aug 12, 2016 at 5:52
-
$\begingroup$ Also, above identity seems incorrect. For example determinant of block graph having $K_5, K_3$ blocks is -10, where from above formula it come out to be 8. $\endgroup$ Commented Aug 12, 2016 at 6:53
-
$\begingroup$ Ops, there was a mistake with sign. Hopefully now it is better. $\endgroup$ Commented Aug 12, 2016 at 9:59
-
$\begingroup$ Thanks for answer. I need some more help, now I want to calculate inverse of its adjacency matrix. Of course it will depend upon ordering of vertices. But for a given ordering can we get some expression for it. ? $\endgroup$ Commented Aug 17, 2016 at 15:47
