Find $x$ that solves $x\left(e^{\frac{a}{x}}-1\right)-y=0$

Question

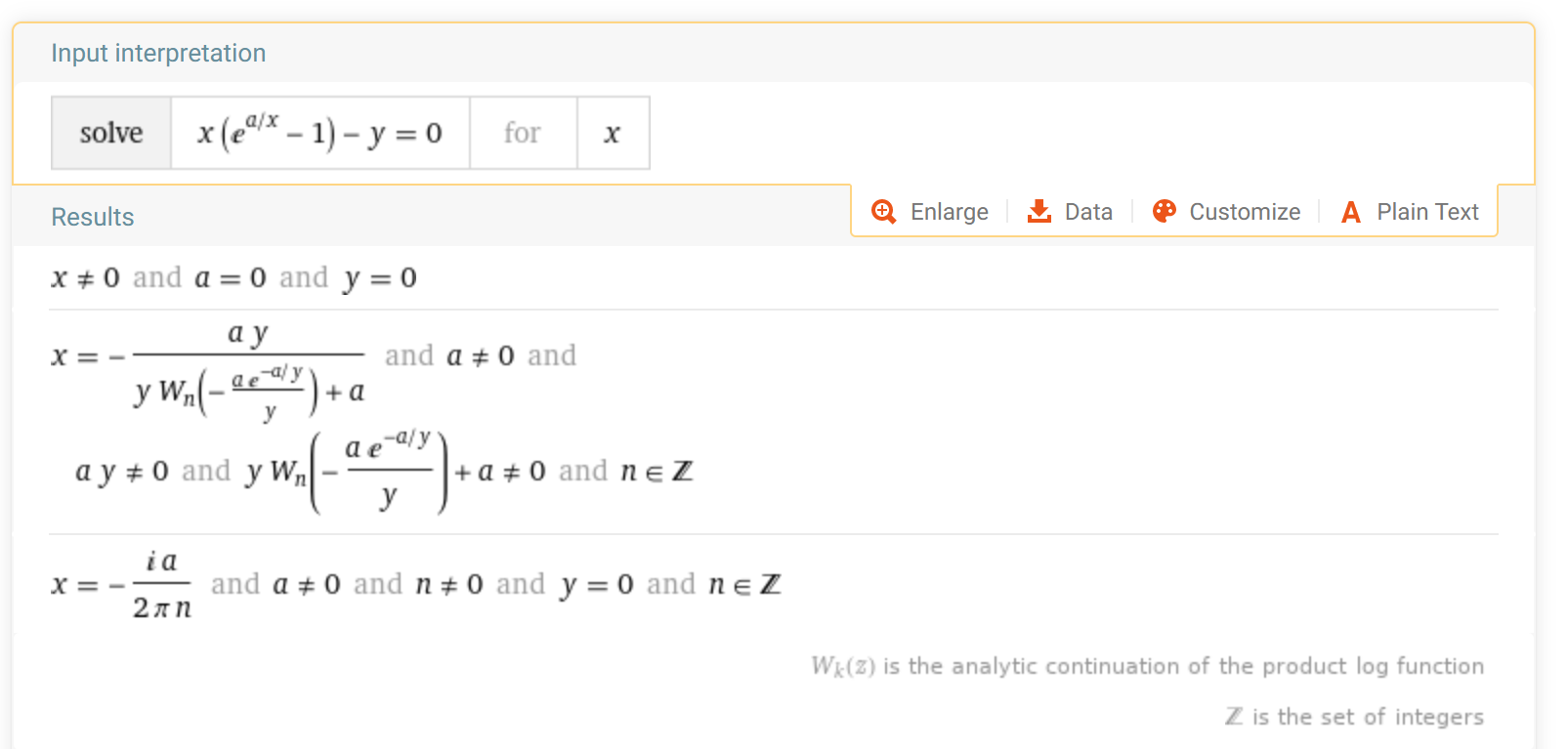
When trying to solve the equation in the title with WA, it produced the following as the solution:

now, if you divide the numerator and denominator by $y$ and set $z:=-\frac{a}{y}$ the solution becomes
$$\frac{a}{W_n(z\,e^z)-z}\ =\ \frac{a}{z-z}\ =\ \frac{a}{0}\ =\ \infty$$

Questions:

• can $x\cdot(e^{\frac{a}{x}}-1)-y=0$ be solved for $x$ and can the solution be expressed with the Lambert $W_n$ function resp.
• can the correctness of the result generated by by WA be explained?

Answer 1

Define $x'=x/a$, $y'=y/a$, then the real-valued solution to $x'(e^{1/x'}-1)=y'$ is $$x'=\frac{-y'}{1+y'W_0(-e^{-1/y'}/y')}\;\;\text{for}\;\;0<y'<1,$$ $$x'=\frac{-y'}{1+y'W_{-1}(-e^{-1/y'}/y')}\;\;\text{for}\;\;y'>1.$$ There is no solution for $y'<0$.
A numerical test: for $y'=1/2$ this gives $x'=-0.6275$ and for $y'=2$ this gives $x'=0.795905$, which indeed solves the equation.

---

The "$\infty$" result at the end of the OP appears because the identification $W_n(ze^z)=z$ is mistaken. (The correct equation is $W_n(z)e^{W_n(z)}=z$.)