1
$\begingroup$

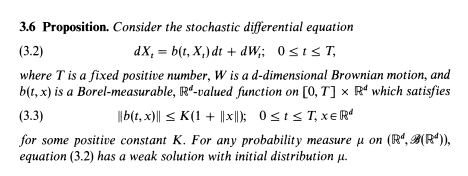
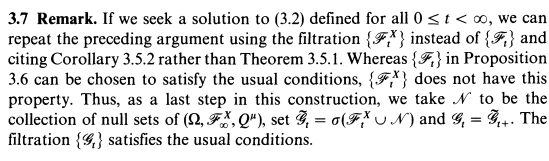
Let $(X, W)$, $(\Omega, \mathcal{F}, \mathbb{P})$, $\{\mathcal{F}_t\}$ be a weak solution to an SDE.

Per definition $W$ is an $\mathcal{F}_t$-Brownian motion and both $X$, $W$ are adapted to $\mathcal{F}_t$. In the typical examples for weak solutions one has $\sigma(B_s | s \le t) \subset \sigma(X_s | s \le t)$, since the Brownian motion is constructed in terms of $X$.

Can I, given a weak solution as above, always replace $\mathcal{F}_t$ by $\sigma(X_s | s \le t)$ and still have a weak solution?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

Almost in some cases and if you have enough regularity to do a Girsanov-type argument. As mentioned in 3.7 Remark in Shreve Karatzas, to get weak solution in enter image description here

we can use the augmented filtration $F_{t}^{X}$ by its null-sets.

enter image description here

However, generally $X$ itself is an unknown so it is hard to make sense of $F_{t}^{X}$ directly. Instead, we start with Brownian motion $(W_{t},F_{t}^{W})$, and to get an $F_{t}$ for which $X_{t}$ is adapted to, we need to augment $F_{t}=\sigma(B_{s},U_{s}:s\leq t)$.

For a concrete construction of this filtration see here https://almostsuremath.com/2010/05/17/sdes-under-changes-of-time-and-measure/ in lemmas 3,4.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .