Lurie's HTT 2.4.1.8

Question

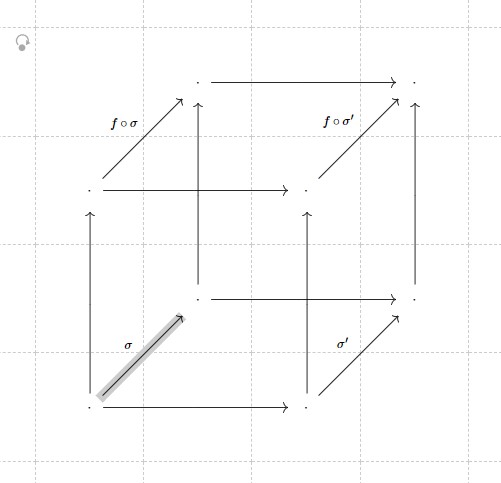
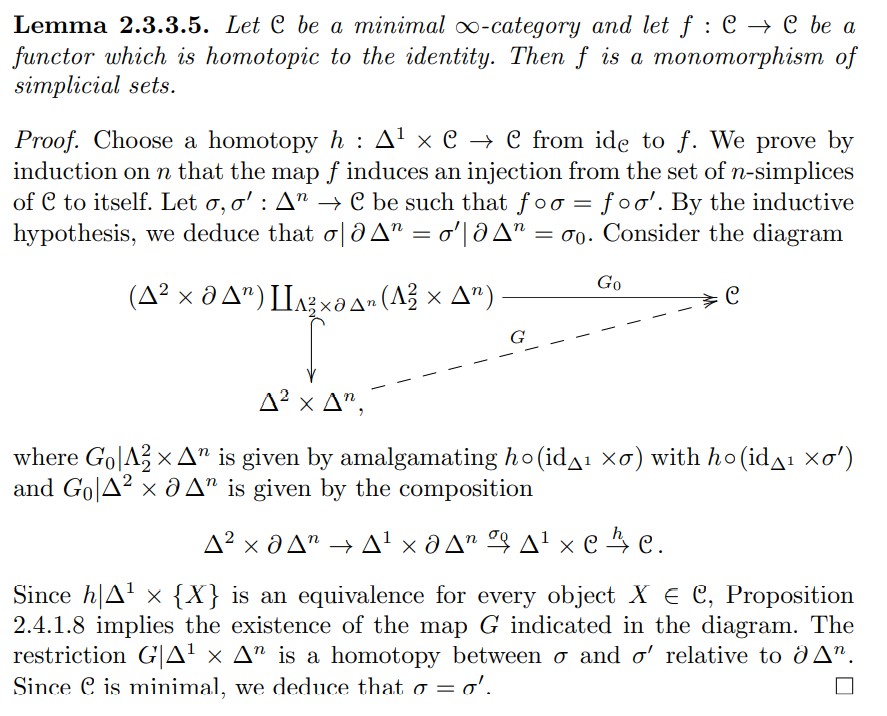
I have some questions about how to apply proposition 2.4.1.8 in HTT which says: 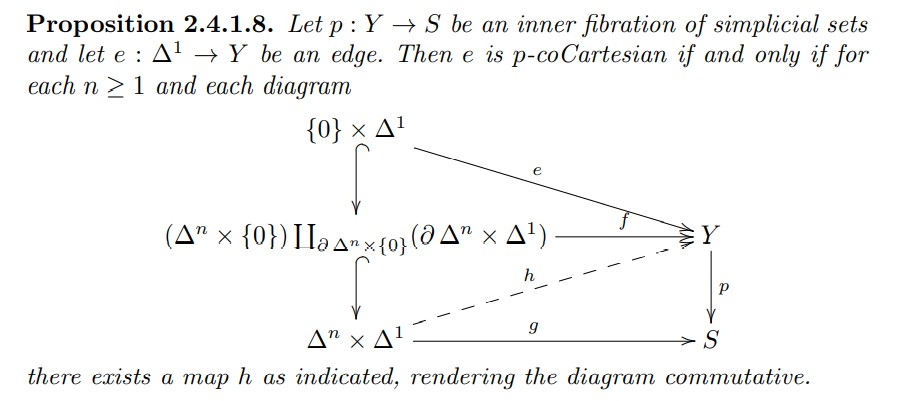In several places, variant forms of this proposition have been used without explanation such as in the proof of Lemma 2.3.3.5: My question is: In 2.4.1.8 we are dealing with the smash product of $\{0\}\rightarrow \Delta^1$ and $\partial \Delta^n\rightarrow \Delta^n$, but in 2.3.3.5 the morphism concerned is the smash product of $\Lambda^2_2\rightarrow \Delta^2$ and $\partial \Delta^n\rightarrow \Delta^n$, How to reduce the case in 2.3.3.5 to the case in 2.4.1.8?

My attempt:we can use 2.4.1.8 to extend $(\Delta^2\times \partial\Delta^n)\coprod_{\Lambda^2_2\times \partial \Delta^n}(\Lambda^2_2\times \Delta^n)\rightarrow C$ as $\partial \Delta^2\times\Delta^1\rightarrow C$.But how can I deal with the interior of $\Delta^2$?

Answer 1

If I understand correctly, this is essentially generalizing the inclusion $\{0\}\to\Delta^1$ in Proposition 2.4.1.8 by an arbitrary left anodyne $B\to B'$ (such as $\Lambda_2^2\to\Delta^2$ in the proof of Lemma 2.3.3.5), namely, solving an extension problem along the inclusion $(\Delta^n\times B)\cup(\partial\Delta^n\times B')\subseteq\Delta^n\times B'$.

The trick (inspired by the proof of 2.1.2.7) is to invoke Proposition 2.1.2.6: it suffices to solve the property under the special case that $B\to B'$ is given by $(\partial\Delta^m\times\{0\})\cup(\Delta^m\times\Delta^1)\subseteq\Delta^m\times\Delta^1$.

Then

\begin{align*} (B'\times\partial\Delta^n)\cup(B\times\Delta^n) &=(\Delta^m\times\Delta^1\times\partial\Delta^n)\cup(\Delta^m\times\{0\}\times\Delta^n)\cup(\partial\Delta^m\times\Delta^1\times\Delta^n)\\&\subseteq\Delta^m\times\Delta^1\times\Delta^n \end{align*}

We swap the second and the third factor in this expression, and this expression is essentially $(\partial(\Delta^m\times\Delta^n)\times\Delta^1)\cup(\Delta^m\times\Delta^n\times\{0\})\subseteq\Delta^m\times\Delta^n\times\Delta^1$, and we need to solve the extension property along this inclusion.

Then the original statement of Proposition 2.4.1.8 seems sufficient.

Answer 2

After playing with some low dimension examples I might know how this is done. e.g the case n=1 in 2.3.3.5 is a consequence of taking n=2 in 2.4.1.8.

Let's draw a cube:

Left face of this cube represents $h\circ (id_{\Delta^1}\times \sigma)$, similar for the right face. The upper face witnesses the fact that $f\circ \sigma=f\circ \sigma'$.

the front-bottom arrow and back-bottom arrows witness the condition on boundaries: $\sigma|\partial \Delta^n=\sigma'|\partial \Delta^n$, which moreover yields the front face and the back face.

Meanwhile, the vertical arrows are all equivalences due to our assumption for $h$, thus we can cite 2.4.1.8 to complete this cube.

It's easy to see this reasoning work for all n not just for n=1.