About Definition 2 in Roĭtman's Paper

Question

Let $A(X)$ be the Chow group of $0$-cycles on a nonsingular irreducible projective variety $X$ over an uncountable algebraically closed field of characteristic zero.

In Definition 2 of Roĭtman's paper _"Rational equivalence of zero cycles" says:

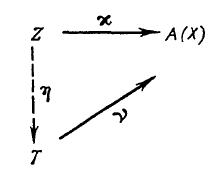
Let $\kappa:Z\rightarrow A(X)$ be a regular map of an irreducible projective variety. Applying Lemma 2 to $Z$ and the subset $ W\subset Z\times Z, W=\{(Z_1,Z_2): \kappa(z_1)=\kappa (z_2)\}$ we obtain

where $\nu:T\rightarrow A(X)$ is a regular map, $\eta:Z\dashrightarrow T$ is a rational dominant morphism and for a c-generic point $t\in T$ the set $\nu^{-1}(\nu(t))$ consists of a countable number of points.

I read many times Lemma 2 and, until now, it is not clear for me which part of Lemma 2 he uses to deduce that:

1. $\nu$ is regular,

2. The set $\nu^{-1}(\nu(t))$ consists of a countable number of points.

Thank you a lot for your help!