Understanding the slice theorem

Question

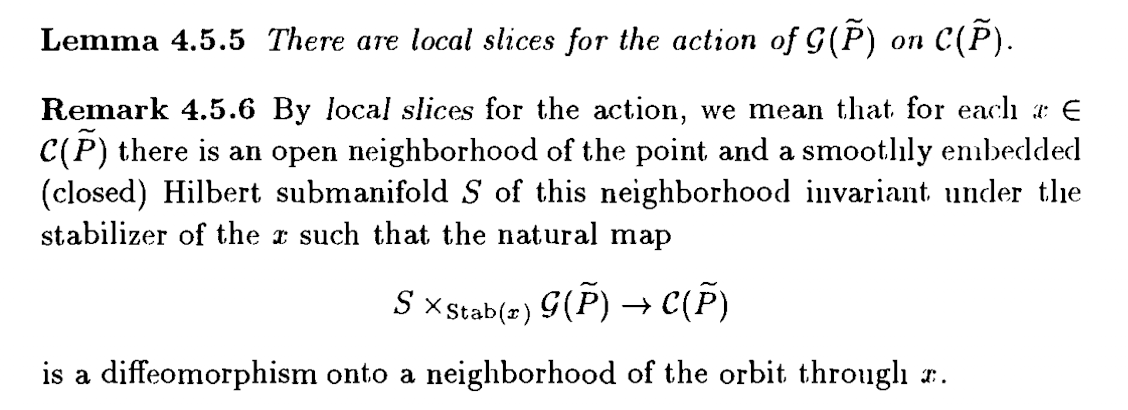
I was reading Morgan's book: "The Seiberg-Witten equations and applications to the topology of smooth four-manifolds" and find it hard to understand the slice theorem (page 62-64).

Here are my questions:

1. The definition of local slice for an action. It states as follows:

I understand every single word of the definition but I do not see how to piece them together. Intuitively, what does a local slice give us?

2. What do we actually get when the group action has a local slice? For example, how do we get for each point, what does the neighborhood look like in the quotient space?

Answer 1

Apart from some technical details, a slice is a (local) submanifold that it transversal to the orbit. For example, in the natural $SO(2)$-action on $R^2$ by rotations, a line segment in the radial direction is a slice.

Concerning your second question, if you have an invariant open neighborhood of an orbit, you get an open neighborhood of that orbit in the orbit space by projection along $M \to M / G$. The existence of slices gives you an open neighborhood of the form $S \times_H G$ so that the projected neighborhood is bijectively identified with $S / H$. Thus once you understand the later quotient (which is easier as $H$ is usually compact), then you can say something about the local structure of the orbit space $M / G$.

A text book reference is for example Duistermaat, Kolk: Lie Groups. As you seem to be interested in the infinite-dimensional case, you may also want to have a look at my paper "Slice theorem and orbit type stratification in infinite dimensions".