Consider the Dirichlet-Laplace eigenvalue problem $$\left\{ \begin{array}{rccl} -\Delta u & = & \lambda u & \text{ in }\Omega\\ u& = & 0 & \text{ on }\Omega \end{array}\right.$$ Suppose $\lambda$ is the smallest eigenvalue and that $\int_\Omega u^2 = 1$.
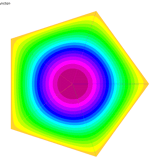
Moreover, suppose that $\Omega$ is a regular pentagon inscribed in the unit circle like shown below. Then the first eigenfunction $u$ looks like this:
For the regular pentagon, consider $T$ the triangle with vertices $(0,0),(1,0),(\cos(2\pi/5),\sin(2\pi/5))$, i.e. slice one fifth of the pentagon. I am interested in computing the quantities: $$ a = \int_T (\partial_x u)^2, b = \int_T (\partial_y u)^2, c = \int_T (\partial_x u\partial_y u) .$$
In fact, it is not difficult to note that because of the symmetry and of the fact that $\int_\Omega |\nabla u|^2 = \lambda$ we have $$a+b = \lambda/5.$$
Also, since rotating by $2\pi/5$ the gradient $\nabla u$ from one "slice" to the next gives the gradient on that slice (because of the symmetry) we may also find that $$ -a\sin (2\pi/5)+b \sin(2\pi/5) +2c \cos (2\pi/5) = 0.$$
Therefore, we have two equations linking $a,b,c$. Finding any other independent relation would give the exact formula for $a,b,c$. However, everything I tried gave me a relation that is a consequence of the two above.
Question: Can we find another relation between $a,b,c$ independent of the two above?
Any help is greatly appreciated!