I want to analyze a special type of weighted directed graph where out degrees is equal to in degrees at each vertex.
$n \in Z$ and $n \geq 3$ ,
Set
$x_n=\left(1,1,\dots,1\right)^T \in \mathbb{R}^{n}$,
and set $$ S_n:=\{A=\left(a_{ij}\right) \in \mathbb{R}^{n \times n} : A^T=-A, A x_n=0,|a_{ij}|\geq 1\ for\ each\ i,j \} $$
then we can define a sequence:
$$ \gamma_n=\inf_{\left(a_{ij}\right)\in {S_n}} {max\left(\{|a_{ij}| \ : 1\leq i,j\leq n\}\right)} $$
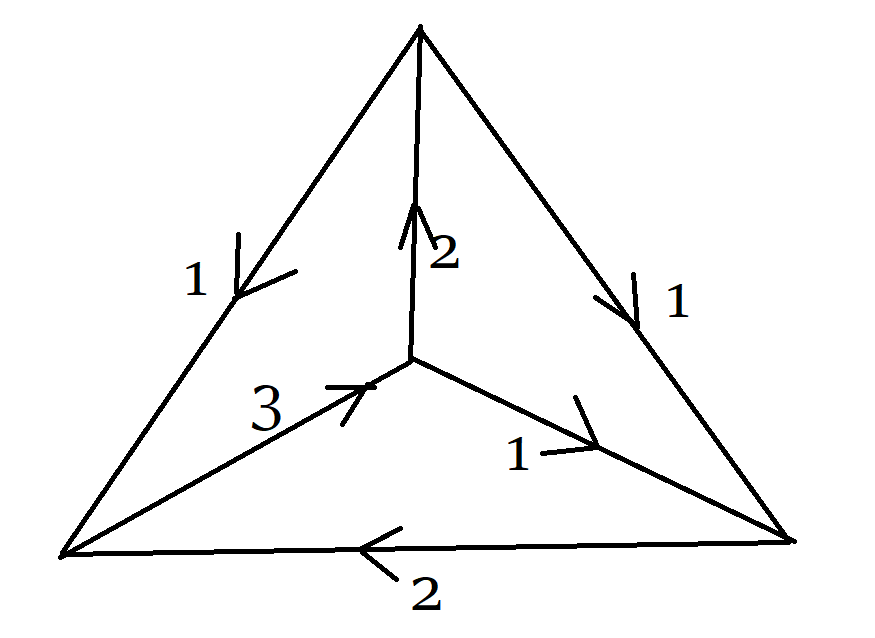
It is easy to prove that $\gamma_{2k+1}=1$, $\gamma_{2k}$ is a decreasing sequence.
It is also easy to prove that $\gamma_4=3$, and I can prove that $\gamma_6=2$ with similar method.I also used computer to calculate that $\gamma_8=5/3$, $\gamma_{10}=3/2$(just numeral calculations but not proof).
Therefore, my first conjecture is the formula of $\gamma_{2k} (k>1)$: $$ \gamma_{2k}=\frac{k+1}{k-1} $$
I have found a method to construct this kind of graph, which means that: $$ \gamma_{2k} \leq \frac{k+1}{k-1} $$
But I cannot improve the lower bound to this formula yet.
2. The second conjecture is more complex(vertices have a varying weight).
define function $\theta$ ( $\mathbb{R}^n \rightarrow \mathbb{R}^n$) as following:
\begin{eqnarray} \left(\theta((a_i)_{1\leq i \leq n})\right)_k= \begin{cases} 1 , & if \ a_k=max(\{a_i\}_{1\leq i \leq n} ) \cr 0, & else \cr \end{cases} \end{eqnarray}
$ Y_k \in \mathbb{R}^n $ is a sequence.
Given a matrix $ A=\left(a_{ij}\right) \in \mathbb{R}^{n \times n} , A^T=-A, A x_n=0$.
If $Y_k$ satisfy that $Y_{k+1}=Y_{k}+\theta(A Y_{k})$
then I guess: $$ \lim_k {\frac{Y_k}{\| Y_k \|}}=\frac{x_n}{\| x_n \|} $$
I have verified it with the the help of computer under many conditions.
It is easy to prove the condition $n=3$, and I think I can prove $n=4$.
In fact, with the help of computer, I guess that that the increasing vertex has a "cycle period"( for large k).With the help of this assumption,I think I can prove the second conjecture for each finite n.
I want to know how can I find similar work.And I am not sure if the first conjecture has been proved cause it's so beautiful.