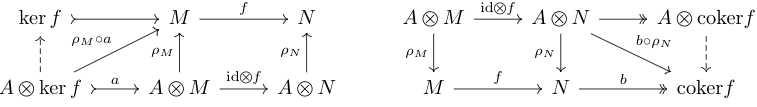Category of modules over internal monoid is abelian

Question

I have asked the following question on MSE a few days ago, but without any success.

I am interested in proving the following statement:

Let $\mathcal{A}$ be a tensor category. Then the category of modules over a commutative monoid $A$ in $\text{Ind}\mathcal{A}$ is abelian and symmetric monoidal, and every such module is the quotient of a flat module.

Here by 'tensor category' I mean a $k$-linear abelian and rigid symmetric monoidal category whose tensor product is $k$-bilinear and exact in both variables. An $A$-module $M$ is flat if the funtor $-\otimes_A M$ is exact.

I am able to show that this category is symmetric monoidal, but I am having some trouble showing that it is abelian and that every module is the quotient of a flat one. What I found out so far:

• The zero object of $\mathcal{A}$ also is a zero object in $A\textbf{Mod}$ together with the trivial action
• As biproduct of $(M,\rho_M)$ and $(N,\rho_N)$ one can just take the biproduct $M\oplus N$ in $\text{Ind}\mathcal{A}$ with the action $\rho_M \oplus \rho_N$
• I think the following diagrams make the kernel and cokernel of a morphism of $A$-modules (taken in $\text{Ind}(\mathcal{A})$) into $A$-modules, hence showing that $A\textbf{Mod}$ has all kernels and cokernels (I think $A\otimes \text{coker} f = \text{coker}(\text{id}\otimes f)$):

• Since the forgetful functor from $A\textbf{Mod}$ to $\text{Ind}(\mathcal{A})$ is a right adjoint of the free functor $X\rightsquigarrow A\otimes X$, it preserves limits and in particular monomorphisms. Therefore a monomorphism in $A\textbf{Mod}$ also is a monomorphism in $\text{Ind}(\mathcal{A})$ and since this is abelian, it is a kernel there. Then since the underlying objects of kernels in $A\textbf{Mod}$ are kernels in $\text{Ind}(\mathcal{A})$, the morphism also is a kernel there.

I have no idea though how to show that every epimorphism is a cokernel in $A\textbf{Mod}$. Also, I do not know how to go about that property with the flatness. I think this is a quite common statement, and yet I could not find a proof anywhere. Any help would be appreciated!

Answer 1

This answer is a summary of the discussion in the comments.

To the question why $A\textbf{Mod}$ is abelian: A morphism in an abelian category is monic (resp. epic) if and only if its kernel (resp. cokernel) is zero. Now suppose we are given an epimorphism $f$ in $A\textbf{Mod}$; then its cokernel is zero, and since the underlying object in $\text{Ind}(\mathcal{A})$ of $\text{coker}(f)$ is the cokernel of the underlying morphism of $f$, we have $\text{coker}(f)= 0$ in $\text{Ind}(\mathcal{A})$ also. Thus, $f$ is epic in $\text{Ind}(\mathcal{A})$ and hence a cokernel. By the construction of cokernels in $A\textbf{Mod}$, this shows that $f$ is a cokernel in this category also. The same works for monomorphisms and kernels.

To the question why every module is the quotient of a flat module: Because the tensor product in $\text{Ind}(\mathcal{A})$ is exact, every free $A$-module is flat. By the unit axiom of modules, the composition

$$ M\simeq I\otimes M \xrightarrow{e\otimes \text{id}_M} A\otimes M \xrightarrow{\rho} M$$

must be the identity on $M$ ($e$ is the unit of the monoid $A$), so in particular $\rho$ is split epic, and hence $M$ is a quotient of $A\otimes M$ viewed as free $A$-module.