Define the unit-stick number $\sigma_1(K)$ of a knot $K$ to be the fewest unit-length
sticks that can realize $K$.
Clearly $\sigma_1(K)$ is at least the stick number $\sigma(K)$.
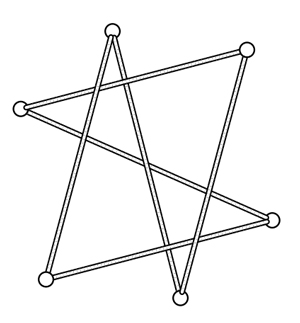
It is known that the trefoil knot $K_{3_1}$ has stick number $6$;
see the image below.
I believe, although I don't have a formal proof, that $\sigma_1(K_{3_1})=6$:
the trefoil also has unit-stick number $6$.

(Wikipedia image by Wiebew. The sticks are not all the same length.)
Q. Is $\sigma_1(K) = \sigma(K)$ for every knot $K$? If not, what is a knot for which it can be proved that the unit-stick number is strictly greater than the stick number, $\sigma_1(K) > \sigma(K)$?
Related: Which knots' stick numbers are twice their crossing numbers?.
Update. User @aorq's comment shows that my "unit-stick number" has been studied under the name "equilateral stick number":
Rawdon, Eric J., and Robert G. Scharein. "Upper bounds for equilateral stick numbers." Contemporary Mathematics 304 (2002): 55-76.
These authors proved that indeed the trefoil has equilateral stick number $6$. They identify the $8_{19}$ knot as possibly one whose equilateral stick number is greater than its stick number. So, about $20$ years ago, it appears that the question I posed was an open problem.