2
$\begingroup$

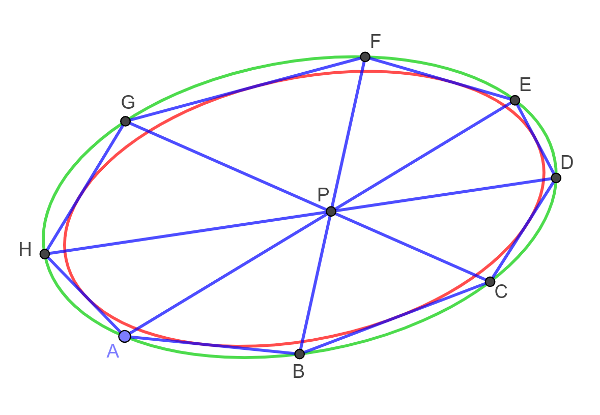
Can you provide a proof for the following claim:

Claim. Given octagon circumscribed about an ellipse. If the vertices of the octagon lie on another ellipse then its principal diagonals meet in a single point.

enter image description here

The GeoGebra applet that demonstrates this claim can be found here.

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ A short proof is as follows: Consider the two ellipses, since there is one octagon inscribed in one and circumscribed about the other, it follows from Poncelet's Theorem that there is a circle of such octagons. Moreover, when one chooses (projective) coordinates so that the inner ellipse $E_1$ is $x^2+y^2=1$ and the outer ellipse $E_2$ is $x^2/a^2+y^2/b^2=1$, by symmetry of the 'translation map', the opposite vertex of the octagon passing through $(x,y)\in E_2$ is $(-x,-y)$, so the line joining them passes through $(0,0)$. QED $\endgroup$
    – Robert Bryant
    Commented May 24, 2021 at 12:09
  • $\begingroup$ By the way, note that the above proof works for any $2m$-gon $P$ that is circumscribed about an ellipse $E_1$ and inscribed in another ellipse $E_2$: The $m$ lines joining opposite pairs of vertices are of $P$ are concurrent. $\endgroup$
    – Robert Bryant
    Commented May 24, 2021 at 13:35
  • $\begingroup$ @RobertBryant Thanks. $\endgroup$
    – Pedja
    Commented May 24, 2021 at 14:06

0

Reset to default

You must log in to answer this question.