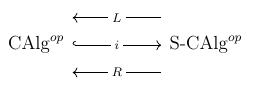
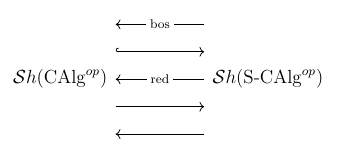Let $\operatorname{CAlg}$ be the category of commutative rings (with unit) and $\operatorname{S-CAlg}$ the category of supercommutative $\mathbb{Z}/2$-graded rings. Then we have an adjoint triple (as usual, right adjoints are below their left adjoints):
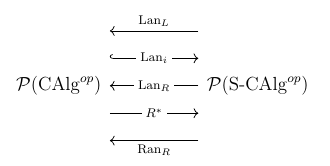
The involved functors are described by taking the even part of a superring with $L^{op}$, the obvious inlusion being $i^{op}$, and dividing the odd part out with $R^{op}$ - we add these peculiar $op$'s for later convenience, note that without them, the order of the adjunctions would be reversed. By taking left and right Kan extensions, this induces an adjoint quintuple
on the presheaf categories - for more on why this is interesting, I recommend this site on the ncatlab where it is developed in the setting of synthetic differential geometry. However, I am trying to figure out how all of this works in the context of schemes and superschemes: As becomes clear using the functor-of-points formalism, the category $\operatorname{Sch}$ is a full subcategory of the (gros) Zariski-Topos, which is itself a full subcategory of the presheaf category on (the opposite category of) commutative rings. A similar construction, of a "Super-Zariski Topos" if one so wishes, is hinted at in here and properly carried out in here - this works more or less as one would expect. However, I found very little on this, does anyone know of a more thorough introduction? By postcomposing with Sheafification and using that composing two adjunctions yields again an adjunction, we obtain an adjoint quintuple between the Zariski Topos and this "Super-Zariski Topos":
It follow from classical references, like the beginning of this survey, that the three functors above restrict to an adjoint triple between Schemes and Superschemes, namely to the operations of taking the bosonic quotient, the inclusion of schemes in superschemes (note that the left Kan extension of a fully faithful functor is fully faithful), and bosonic reduction. My main question is the following: Do the other two functors at the bottom restrict as well? Schreiber indeed uses them in the theory of superformal smooth sets or more generally solid topoi, but are they also of any use in the algebraic geometry of superschemes (even if they might only live on these bigger topoi)? And is there a reference that gives more classical, or at least more concrete constructions for them (this seems pretty difficult to me since this relies, for the upper three, on properties of left Kan extensions)?