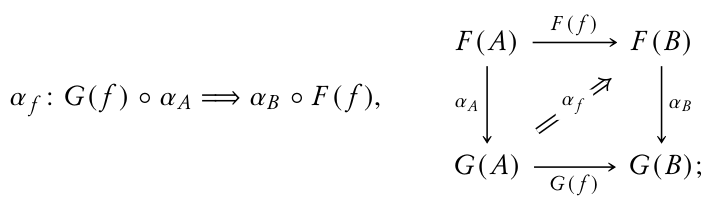The bicategorical Yoneda lemma (see [Johnson–Yau, Chapter 8]) states that, given a bicategory $\mathcal{C}$ and a pseudopresheaf $\mathcal{F}\colon\mathcal{C}^{\mathsf{op}}\to\mathsf{Cats}_{\mathsf{2}}$, we have an equivalence $$ \mathsf{PseudoNat}(\mathsf{h}_{(-)},\mathcal{F}) \cong \mathcal{F} $$ in $\mathsf{PseudoFun}(\mathcal{C},\mathsf{Cats}_{\mathsf{2}})$. If one replaces $\mathsf{PseudoNat}$ by $\mathsf{LaxNat}$, then one doesn't have an equivalence anymore, but rather, for each $A\in\mathrm{Obj}(\mathcal{C})$, an adjunction $$ \mathsf{LaxNat}(\mathsf{h}_{A},\mathcal{F}) \rightleftarrows \mathcal{F}(A) $$ (see [nLab, lax natural transformation]).
Now, recall that, given
- A bicategory $\mathcal{C}$;
- A subclass $E$ of the class $\mathrm{Mor}_{1}(\mathcal{C})$ of $1$-morphisms of $\mathcal{C}$ such that, for each $A\in\mathrm{Obj}(\mathcal{C})$, we have $\mathrm{id}_{A}\in E$;
- A pair of parallel lax functors $F,G\colon\mathcal{C}\rightrightarrows\mathcal{D}$ from $\mathcal{C}$ to another bicategory $\mathcal{D}$;
we define an $E$-lax transformation (see Sigma limits in $2$-categories and flat pseudofunctors and Bilimits are Bifinal Objects) from $F$ to $G$ as a lax transformation $\alpha\colon F\Rightarrow G$ such that, for each $1$-morphism $f\colon A\to B$ of $\mathcal{C}$ in $E$, the lax naturality constraint
of $\alpha$ at $f$ is invertible.
As examples, if $E=\{\mathrm{id}_{A}\}_{A\in\mathrm{Obj}(\mathcal{C})}$, then $E$-lax transformations agree with lax transformations, and if $E=\mathrm{Mor}_{\mathsf{1}}(\mathcal{C})$, then $E$-lax transformations agree with pseudonatural ones.
Question: What is the smallest marking on $\mathcal{C}$ for which an "$E$-marked lax bicategorical Yoneda lemma" holds? I.e. such that we have an equivalence $$ \mathsf{LaxNat}^+_E(\mathsf{h}_{(-)},\mathcal{F}) \cong \mathcal{F} $$ in $\mathsf{PseudoFun}(\mathcal{C},\mathsf{Cats}_{\mathsf{2}})$?