Is the union of two proper flows proper?

Question

Let $\varphi _t$ be a flow, aka. a one parameter group of homeomorphisms of the open subset $\Omega \subseteq {\mathbb R}^n$, which we assume to be continuous in the usual sense that $$ (t, x)\in {\mathbb R}\times \Omega \mapsto \varphi _t(x)\in \Omega $$ is jointly continuous.

One says that $\varphi $ is proper when the map $$ \Psi :(t, x)\in {\mathbb R}\times \Omega \mapsto \big (\varphi _t(x), x\big )\in \Omega \times \Omega $$ is proper, meaning that the inverse image under $\Psi $ of every compact set $K\subseteq \Omega \times \Omega $ is compact.

Properness may also be characterized by the fact that if $\{x_n\}_n$ is a sequence in $\Omega $, converging to some $x$ in $\Omega $, and $\{t_n\}_n$ is a sequence in ${\mathbb R}$, such that $\varphi _{t_n}(x_n)$ converges to some $y$ in $\Omega $, then the $t_n$ are bounded.

---

For $i=1,2$, assume that $U_i$ is an open subset of $\Omega $, invariant under $\varphi $, and such that the restriction of $\varphi$ to $U_i$ is a proper flow.

Question. Is the flow restricted to $U:= U_1\cup U_2$ proper?

As far as I can see the proof should start as follows: let $\{x_n\}_n\subseteq U$ converge to some $x\in U$, and such that
$$ y_n:=\varphi _{t_n}(x_n)$$ converges to $y\in U$.

If both $x$ and $y$ lie in $U_1$, or if both $x$ and $y$ lie in $U_2$, the conclusion is immediate. So assume that $x\in U_1\setminus U_2$ and $y\in U_2\setminus U_1$. It follows that $ x_n\in U_1,$ and $ y_n\in U_2,$ for all sufficiently large $n$, so we conclude that $x_n$ and $y_n$ eventually lie in $U_1\cap U_2$ by invariance.

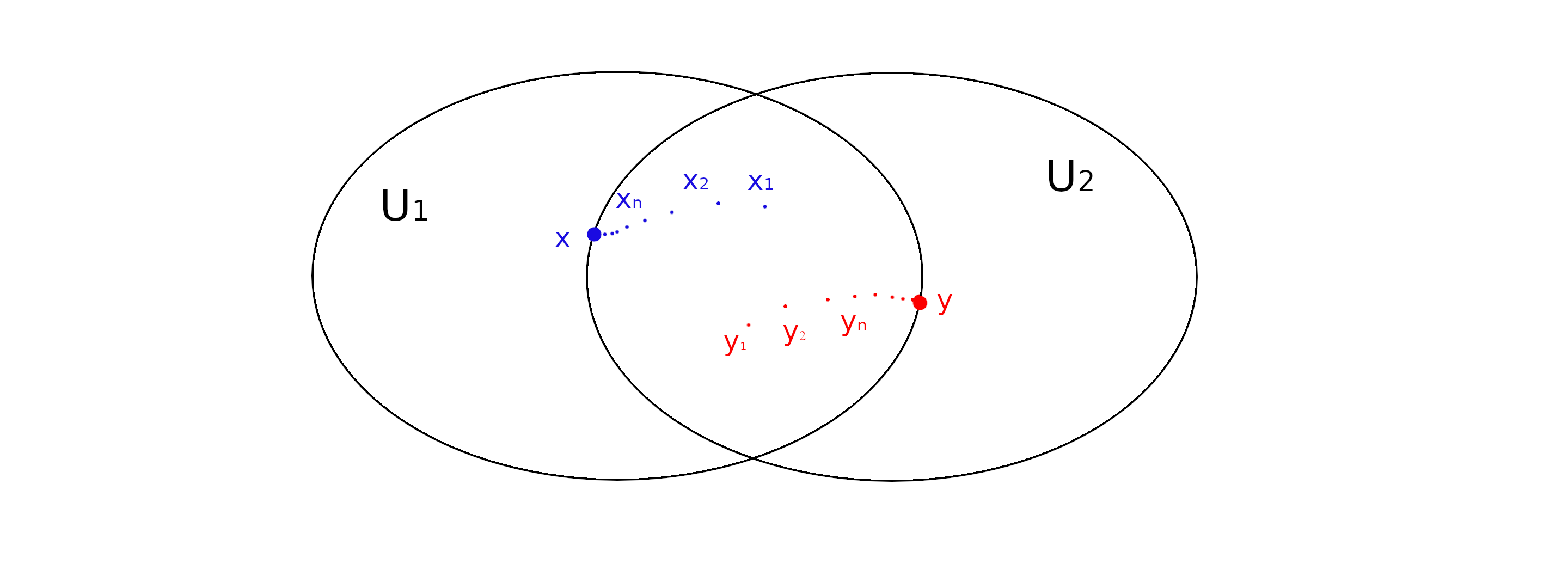Therefore $x$ is in the boundary of $U_2$, and $y$ lies in the boundary of $U_1$.

Can't see where to go from here... After struggling with it for a few hours I am beginning to feel like it might be false :-(

Answer 1

Consider the flow on $\Omega={\mathbb R}^2$ given by $$\varphi_t(x,y)=(e^tx, e^{-t}y).$$ Take $U_1$ equal to the open upper half-plane ($y>0$) and $U_2$ equal to the open right half-plane ($x>0$). I leave it to you to verify that the flow is proper on both $U_1, U_2$ and is not proper on their union.

---

Edit.

1. The flow is proper on the open right half-plane because it is conjugate to the linear flow $$ \psi _t(x, y) = (x+t, y), $$ on ${\mathbb R}^2$, via the diffeomorphism $$ (x, y)\in (0, +\infty )\times {\mathbb R}\ \mapsto \ \big (\ln(x), xy\big )\in {\mathbb R}^2. $$ A similar reasoning implies properness of the flow on the open upper half-plane.

2. The flow is not proper on the union $U=U_1\cup U_2$ because the sequence $\{v_n\}_{n\in {\mathbb N}}\in U$, given by $$ v_n=(e^{-n}, 1), $$ converges to $(0,1)$, while for $t_n=n$, we get $$ \varphi_{t_n}(v_n) = (1, e^{-n}) $$ which converges to $(1,0)\in U$ as $n\to\infty$.