What is the paper where the Liouville theorem for harmonic function was first stated? Did it come before or after (or in the same paper) as the Liouville theorem in complex analysis?
1 Answer
$\begingroup$
$\endgroup$
4
References to Liouville go back to his 1847 result that a doubly periodic function without poles is identically constant, which does not yet contain the generalization to either harmonic functions or holomorphic functions.
I quote from Barry Simon, Harmonic Analysis: A Comprehensive Course in Analysis, Part 3 (page 197):
That any positive harmonic function is constant is due to Bôcher (1902), although the theorem is often named after Picard’s rediscovery (1923) — there is often reference to the Liouville–Picard theorem.
Bôcher states the theorem in a footnote:
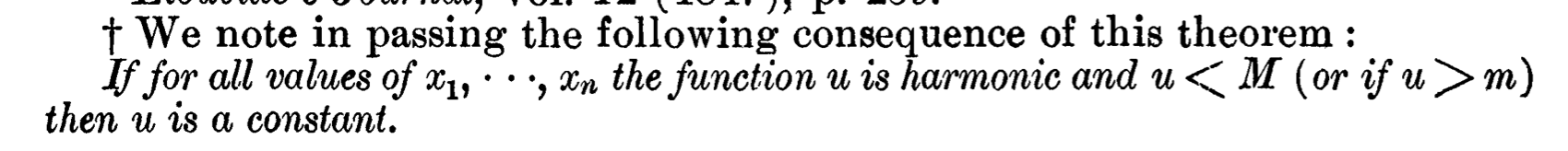
J. Liouville, Leçons sur les fonctions doublement périodiques (1847).
M. Bôcher, Singular points of functions which satisfy partial differential equations of the elliptic type, Bull. Amer. Math. Soc. (2) 9, (1903), 455–465.
É. Picard, Deux théorèmes élèmentaires sur les singularités des fonctions harmoniques, C. R. Acad. Sci. Paris 176 (1923), 933–935.
answered Jan 10, 2021 at 12:52
-
$\begingroup$ Thank you! What is the relationship between doubly periodic and holomorphic functions (for which the complex Liouville theorem is usually stated)? $\endgroup$– user140746Commented Jan 10, 2021 at 15:16
-
$\begingroup$ @Zyl --- here it is explained that the doubly periodic function is bounded, so it is a special case of a bounded holomorphic function. $\endgroup$ Commented Jan 10, 2021 at 15:20
-
$\begingroup$ Ok, thanks! What about the more general result on holomorphic functions? Is it in another paper by Liouville? $\endgroup$– user140746Commented Jan 10, 2021 at 15:31
-
$\begingroup$ the holomorphic result was first announced by Cauchy, in 1844, in response to Liouville's announcement of his doubly periodic result, see this book $\endgroup$ Commented Jan 10, 2021 at 19:29