Given a ball $B$ and a linear subspace $L$ in $\mathbb{R}^n$, what is the maximum value of $\frac{vol(B \cap C)}{vol(C)}$ where $C$ is a cube of the form $x + [0, h]^n$ for $x \in L$ and $h \in \mathbb{R}_{\geq 0}$?
The above ratio has an interpretation as the probability of a randomly chosen point (with respect to the uniform distribution) on the cube falling in the ball. The answer is $1$ if $L \cap interior(B) \neq \emptyset$, so you can assume that $L \cap interior(B) = \emptyset$. The general solution seems to be hard for a fixed cube $C$ (related questions: this, this, and this), but I am hoping the optimization problem (where the end point $x$ and the length $h$ are both variable) would be easier (say by some geometric argument).
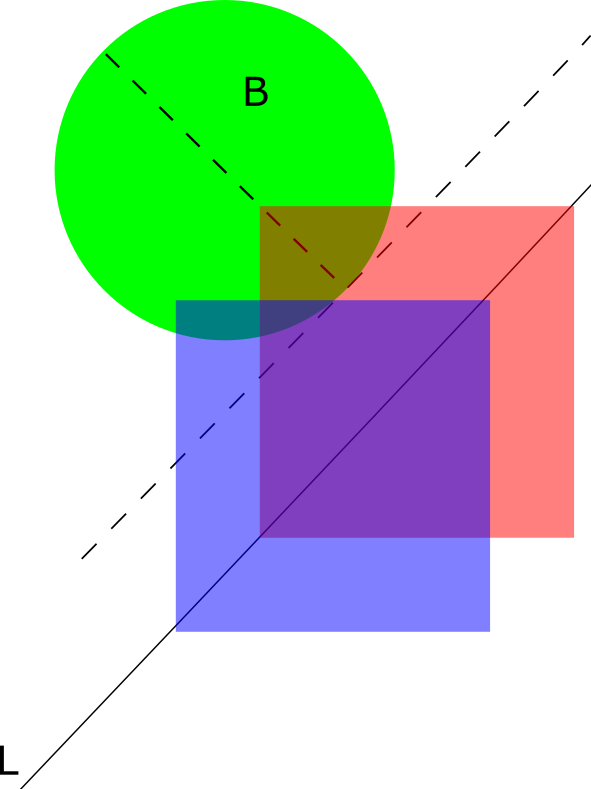
Even the following special case would be interesting: $n = 2$ and $L$ is the "diagonal" line, i.e. the line through the origin and $(1,1)$. In this case $C$ is a square, and my conjecture is that in the optimal case the diagonal of $C$ which is not along $L$ will pass through the center of $B$. More specifically, in the figure below the ratio for the red square would be higher than the ratio for the blue square (where both squares have the same width). If this is true, this would decrease the dimensionality of the optimization problem by one.